71. From Barley Corns to the Speed of Light
- M Campbell
- Mar 23, 2024
- 24 min read
Updated: Apr 12, 2024
The metre, symbol m, is the SI unit of length. It is defined by taking the fixed numerical value of the speed of light in a vacuum c to be 299792458 when expressed in the unit m⋅s−1, where the second is defined in terms of the caesium frequency ΔνCs.

This is most recent official definition of the metre, from 2019. The metre was first defined in relation to the speed of light in 1983. Defining a unit of length in terms of a motion, or a speed, which itself depends on a unit of length, is circular, so why was the metre defined in this way? Since the inch is defined in relation to the metre, which is 10 000 / 254 inches, the inch is also dependent on this definition. In the context of light defining units of measure, what is the meaning of 254? In the past, many systems of measure relied on elements of nature as standards, in particular the barley corn. While the barley corn had its drawbacks, being slightly variable in size, how much better is it to attach a system of measure to a speed instead?
1. Circularity
It is difficult to find a natural standard against which to define a basic unit of measure. Obviously, feet and arms vary in size. And measuring the earth's meridian or equator is not a straightforward exercise, because it is not only very big, by human standards, but it does not have a smooth and uniform surface. Also, since it's not a perfect sphere, but bulges at the equator, you have to measure the entirety of the distance between the equator and the pole to get a measure for the earth's circumference, rather than just a small section. This gives rise to many possible measurements. Light seems to provide the solution to finding a reliable, durable standard, since it is believed to move at a constant speed. Yet, this is not a universally accepted theory. Moreover, the metre (and the inch) aren't measured in terms of a linear, spatial distance, but in terms of a speed. Speed is typically defined as the rate of motion of an object, and it is measured as the distance traveled per unit of time. Mathematically, speed is calculated as "distance traveled" divided by "time taken", since speed depends on how far an object travels over a certain period of time. The circularity of trying to define a unit in terms of the speed of something that was so relative to distance in the first place does seem to undermine the validity of the metre, as the fundamental unit of our age. Linear units, such as metres or inches, are measures of distance in space, and while they exist in time, they need not depend on any speed. The measure of a speed depends on there being a measure of space in the first place, as well as a on a measure of time.
How were standards of measure arrived at historically?
2. From louse eggs to space
By contrast, ancient systems of measure had tangible, easy to visualise (if not always easy to see) standards from the natural world, which didn't involve any concept of speed. It is sometimes thought that these standards were imprecise because many units in the past were called things like "foot" or "span" or "digit", seemingly implying an actual, mortal, changeable human as the ultimate standard. However a close study of ancient units of measure shows a high degree of precision, that are part of well designed systems. Various kinds of feet - English, Belgic, Persian, Egyptian, etc. - existed as part of a single over-arching system, as has been demonstrated by various researchers, including John Michell and John Neal, Jim Alison, Stephen Dail and David Kenworthy. And while feet make good visual references for a unit of around 12 inches, no individual person's foot is likely to ever have been the basis of this system.
Most ancient units reveal simple numerical relations to other units, sometimes fractional, sometimes irrational, from which very small and very big lengths can be arrived at. The old English system illustrates this: the moon's equatorial radius, for example, is 1080 miles, and 1080 is an important number in ancient astronomy and metrology, also associated with time divisions, for example in the Hebrew calendar. The inch is three barley corns, and the number 3 is also an important number traditionally. Between these two scales, and beyond, a whole organic system stretches out, like mycelium, linking aspects of the natural world. It is based mostly on 3s and 4s, and the geometries of the square and circle.
In the ancient Indian tradition, as explained in the Brahmanda Purana, 8 Paramānus (परमानु), or atoms, make a Trasarenu (त्रसरेणु) (1), or pollen dust, 8 Trasarenu make 1 Ratharenu (रथरेणु, 8 Ratharenu are a Balāgra (बलाग्र), 8 Balāgra make a Likṣā (लिक्षा), a louse egg, 8 Likṣā (2) make a Yūkā (यूका), a louse, 8 Yūkā make 1 Yava (यव), a barleycorn, 8 Yavas make 1 Aṅgula (अङ्गुल)(3), or finger-breadth. The following extract shows how the system goes from atomic measure to astronomical measure:
114. On hearing their utterance full of humility, Mātariśvan (the Wind-god) uttered the following sweet words (explaining everything) in the proper order and in the manner seen by him.
Vāyu said
116. There are ten parts of the Mahat. The gross part is called Bhūtādi (i.e. Ahaṃkāra or Cosmic Ego). The magnitude of Bhūtādi is more than a tenth part (?).
117-118. Paramānu (Atom) is very subtle. It can be mentally conceived but it cannot be seen by the eyes. What cannot be further broken up in the world should be known as Paramānu (atom). When the rays of the sun come through the window, minute dust particles are seen. The learned men call that particle a Paramānu. It is the first in the series of units of measurement.
119-120. When eight Paramānus join together it is said to be of the size of the pollen dust of the lotus (Padmarajas) and it is called Trasareṇu.
The combination of eight Trasareṇus is proclaimed as Rathareṇu. The combination of eight such (Rathareṇus) is remembered as Bālāgra (hair-tip) by the learned men.
126. Two thousand Dhanus units make one Gavyūti. Eight thousand Dhanus units make one Yojana.
127-128. The measurement of the distance of a Yojana is based on this unit of Dhanus. It should be known that a thousand, of these Dhanus make the distance called Śakrakośa[8] (?) It has been reckoned so by the experts in the knowledge of calculation. Listen to the distances of worlds in the creation of Brahmā with this Yojana as the unit.
129. The sun is more than a hundred thousand Yojanas from the surface of the Earth. The moon is two hundred thousand Yojanas above the Sun. (4)
This system goes from the very small, the atom, to the very large, the sun's distance from earth, and the "cosmic ego". It shows that from ancient times, it has been possible to think in terms of the very small and the very big with some precision, and without reference to speed as a standard.
The atom referred to here is not quite the same as what it has come to mean in modern times, it is a theoretical unit that derives from a logical progression, of dividing into ever smaller parts, until the smallest part that can be imagined is arrived at. The atom is called Paramānu, and cannot be subdivided any further, and is considered eternal. It's not a bad basis for a system of measure, though it is unclear whether such a thing was in fact physically measured, or simply inferred. Atoms have characteristic sizes, and their size is a fundamental property of each element and is considered constant under typical conditions. Atoms could still, in theory, provide a basis for a system of measure, even though they are not the smallest building blocks of matter.
3. Barley corns

Many traditional systems feature the barley corn, as a standard both of length and of weight. The barley corn is a more attractive proposition than the louse egg, and easier to measure than the theoretical, intangible atom, which, in the ancient systems at least, seems to have been basically imaginary. There are problems with measuring seeds of course, as they can vary in size, and different species have variations too. The traditional English system of measurement, before it was replaced with the imperial, was based on the barley corn. Three barley corns laid end to end were defined as one inch, and twelve inches made up one foot. The inch has only changed very slightly since then. A 14th century English law defined the inch as “three grains of barley, dry and round, placed end to end lengthwise”. A 10th century Anglo-Saxon document, ‘PAX’, defined the bounds of the ‘King’s Peace’ from his residence as "3 miles, 3 furlongs, 3 acre breadths, 9 feet, 9 shaftments and 9 barleycorns”. Similar to the English system, the Scottish system of measurement also used barley corns as a basis for defining units of length. Three barley corns were defined as one nail, sixteen nails made up one ell, and so on. The Irish system of measurement also used barley corns as a unit of length. Three barley corns also made up one inch. The barley corn was also associated with units of weight and volume.
Curiously, the barley corn can also be associated not only with space, as a linear unit, and as a unit of weight, but also with time, in the Hebrew calendar, as Irv Bromberg explains:
The time degree also very nearly equals the difference in duration between the solar day and sidereal day, which in the present era amounts to about 3 minutes and 55.9 seconds. The Babylonian finger was 6 barleycorns = 1/12 of a time degree = 1/3 of a minute = 20 seconds of time. The cubit was 180 barleycorns = 5/2 time degrees = 10 minutes of time.(4)
However, it's one thing for a unit to be linked to space as well as time, and another for a unit to be defined in terms of space and time simultaneously, as the metre is. In 1930-31, at Mohenjo-daro, Ernest Mackay discovered a broken piece of shell bearing 8 divisions of 6.7056 mm each, which is equivalent to 0.264 inches, which multiplied by 8 is 2.112 inches. With pi as 25/8, in keeping with the 8 divisions, a diameter of 8 divisions of 6.7056 mm, or 0.264 inches, which is 4 Angulas, gives a circumference of 10 divisions of 16.764 mm, or 0.66 inches, which is 10 Angulas. 96 Angulas make an ordinary Dhanusha, and this is exactly a thousandth part of an English mile. A Yojana of 8000 Dhanusha (of 108 Angulas each) is 9 miles and 1000 Dhanushas of 96 Angulas each are 1 mile. The Indian measures coincide with many other units, from the Saxon wand of 39.6 inches, which works out at 150 x 0.264, the Saxon foot of 13.2, equivalent to 50 x 0.264, and the Sumerian shusi, as found on the statue of Gudea, of 0.66, which relates to 0.264 as 5/2.
In his article for the Encyclopedia Britannica, Flinders Petrie settles on an old foot of 13.22 inches for England. Jim Wakefield found the a foot of 13.2 inches fitted in the circle of the Rollright Stones in England. (12) Flinders Petrie does however also describe a 13.3 inch foot, which would imply a barley corn of nearly 1/3 of a modern inch. (6)
Barley corns were once an important component of systems of measure. The shusi as we know it from the shell at Mohenjo-Daro and the statue of Gudea would have measured 0.66 modern inches. (16)
4. Reading ancient sites in terms of metres
So how did we end up with a worldwide system of measure that is defined in a circular way in relation to itself, and which seemingly has nothing to do with the traditional systems which relied on physical entities such as the barley corn? Is the metre a complete travesty, compared to the sophisticated systems of measure that have come down to us through the centuries and which existed in many different parts of the world for so long? Many people seem to think so. One of the reasons for this is that the system that the various kinds of feet and cubits were part of was so well designed. It is only to the credit of its designers that the metre fits so well within it. The current system that has been built around the metre is less sophisticated, in many ways, but we should not hold it against the metre, which is much older.
One indication that the metre may actually have a place not only within this tradition is that we find it, or something close to it, in ancient sites, from the Giza pyramids, to stone circles in Brittany, to Stonehenge. By that, I mean that reading dimensions taken from these sites gives meaningful numbers. So the side of the Great Pyramid of 9068.8 inches, as per Flinders Petrie (11), converts to 230.34752 metres, this is very close to being 12² x 4² / 10 metres, a possible connection to a sexagesimal system such as the Babylonians, among others, used.
We can also interpret the side of the Great Pyramid as √2/√3 x 100 000 / 354.36708 = 230.40983 metres, equivalent to 9071.2531 inches. A lunar year is 354.36708 days. An inch is 2.54 cm. The existence of the metre owes a lot to the number 254, in its role with the inch as "day-inch". A lunar year can be represented geometrically as 200 000 x √2 / (254 x π) = 354.45524, which basically means that 20 x √2 /π metres are very nearly equal to a lunar year in day-inches. This is especially interesting when 20 cm x 2.61803, which is Phi squared, make a royal Egyptian cubit, as 52.3606 cm (20.6144 inches), and also, if we were to add a value of 2.61803 cm to this cubit in cm, we would obtain a nice approximation of pi, 3.141636. Furthermore, if we were to multiply that cubit as a value in inches by the number of days in a lunar year and divide by 20, we would obtain a nice approximation of the solar year in days: 20.6144 x 354.36708 / 20 = 365.2534. This gives a strong indication that the metre and the inch are both intimately connected to the Giza plan, and to each other.



The number 254, which links inch and metre, is also found as a ratio at Giza.

There are several examples of measures with lunar associations in metres at Giza. Here is one found by Dennis Payne (15):

Howard Crowhurst has found reading dimensions and distances in metres yielded significant results in Brittany, and Quentin Leplat has found evidence for the metre in ancient times. Richard Heath and Robin Heath have worked with the possibility in Brittany that there is a metre at work in the design of stone circles, equivalent to 29.53059 x 4/3 inches. In the context of the repeated use of the "day inch", this is significant. Richard Heath has written that "the practice of day-inch counting leading to a definition of the megalithic yard (32 and 5/8 inches) from the relative lengths of lunar and solar years, that is as a natural metrological consequence of counting days in inches."(10)


5. The metre is compatible with ancient units of measure
Can the metre be attached to the ancient traditions that were based on things like lice, barley corns and finger breadths? If a barley corn measures a third of an inch, 29.53125 x 4 barley corns measure 39.375 inches, a form of a metre. And 29.53059 x 4 barley corns make 39.37412 inches.
It is not easy to assemble 29.53059 barley corns, but this measure can be achieved with a circle. A circle with a diameter of 94 units will have a circumference of 295.309709 units, which is close to 10 lunations in days. This was discovered by Jim Wakefield, at the Rollrights Stone circle. (12) If we lay out 376 barley corns in a line and draw a circle around it, so that this line is the diameter, the circumference will be 1181.2388 barley corns, equivalent to 40 x 29.530971 barley corns, and to 10 x 39.3746279 inches, which is approximately 10 metres.
The Megalithic Yard is a unit associated with stone circles, discovered by Professor Alexander Thom, which he valued at 2.72 feet +- 0.003. In terms of the geometry of circles and triangles which Thom found in his work, the Megalithic Yard can be usefully described as 2 π / √3 x 9 inches = 32.6484 inches (13). In terms of a possible sexagesimal system in which a unit close to the metre existed once, it's also interesting to think of the Megalithic Yard as 12⁴ x 4 / 10⁵ metres, or 12⁴ x 4 / 10⁵ x 1/254 inches. This would be compatible also with the Great Pyramid side multiplied by 36 / 10 000.
9068.8 x 36 / 10 000 = 32.64768
32.64768 / 12 = 2.72064
Since the side of the Great Pyramid can be interpreted as 5000 π / √3 inches, we can then say that a Megalithic Yard is also compatible with 18 π / √3 = 32.6483886 inches, which make 2.720699 feet.
We could interpret the Megalithic Yard, theoretically, as 54 x π / √3 barleycorns of 1/3 inch, which is interesting in the context of 54 being an important number in ancient metrology and Indian astronomy, and half of 108.
54 x π / √3 x 1/3 = 32.648389
32.648389 / 12 = 2.720699
If we can also think of the Megalithic Yard as 48³ x 29.53059 / 10⁵ inches, that's equivalent to 12⁴ x 4² x 29.53059 / 10⁵ barley corns of 1/3 inches.
12⁴ x 4² x 29.53059 / 10⁵ x 1/3 = 32.65847
32.65847 / 12 = 2.721539
We could return to the circle, and express the Megalithic Yard as 376 x 12⁴ x 4 x π / 10⁶ barley corns.
376 x 12⁴ x 4 x π / 10⁶ x 1/3 = 32.658891
32.658891 / 12 = 2.721574
Or we could express it as 47 x 4⁶ x 9² x 2 x π / 10⁶ barley corns.
47 x 4⁶ x 9² x 2 x π / 10⁶ x 1/3 = 32.65889
There are many connections between ancient units and the metre. As Jim Alison points out:
One meter contains 60 shusi, or 54 digits, or 30 Indus Valley inches. The length of the Babylonian foot of 20 shusi, the old Northern foot of 18 digits and the Indus Valley foot of 10 Indus Valley inches, are all one-third of one meter. (17)
Other indications that the metre may be very old is that we find ancient units of measure that seem to be compatible with it, though often it is hard to know if the information has simply been rounded to an integer by someone who likes to work in metres anyway. There are possible traces of an ancient metre as far away as China:
The territory was divided into squares which had a li furlong or three hundred feet on each side, and contained nine hundred square meters. The length of the pou was six tch'eu, and that of the tch'eu was approximately twenty centimeters. Therefore, the louse was approximately one meter twenty centimeters, the stadium 360 meters, the square stadium 129,600 square meters, and the meou 144 square meters or a little less than an are and edmi"
Flinders Petrie did not work in metres, but presented his work mostly in inches. He wrote in 1911:
It is remarkable how near this early decimal system of Germany and Britain is the double of the modern decimal metric system. Had it not been unhappily driven out by the 12-in. foot, and repressed by statutes both against its yard and mile, we should need but a small change to place our measures in accord with the metre. (6)
Surprisingly, according to Flinders Petrie, Britain, and northern Europe, once had a decimal system of measure, and a unit close in size to the modern metre. He writes about an ancient foot of 13.22 inches, which was in England "the commonest building foot up to the 15th century"(7). What remains of the predecessor of the imperial system in Britain is indeed decimal: a mile is 8 furlongs, but was once 10 furlongs. A furlong is 10 chains, a chain is 10 fathoms. 400 feet of 13.2 inches fit into a mile, and this foot of 13.2 inches gives a yard of 39.6 inches, which is 54 x 0.733333 inches. A unit of about 40 inches was in use, called the yard and full hand, and a third of that would have been approximately 13¹/³ inches.
If we multiply 40 inches by 63 / 64, the Eye of Horus fraction, we obtain 39.375 inches, an ancient form of metre, and this multiplied by 8000 / 8001 gives the modern metre of 10 000 / 254 inches.
The Saxon / Northern foot was approximately 13.2 inches, and is also found in Sumeria and India. The Indian system is in part compatible with a decimal system, from the Dhanus up. In the Brahmanda Purana, from the atom upwards, every subsequent unit in the system derives from a multiplication by 8, seven times, until the angula, the finger breadth. From the angula, the system progresses not by 8s but by a different numbers: 12, 21, 24, 42, and 96, until the Dhanus, which themselves are counted in hundreds and thousands, for example 300, 1000, 2000, 8000.
The metre fits in with the ancient system of measure. The ancient is connected to the system of feet and cubits sometimes as 10 000 / 254 inches, and sometimes as 29.53059 x 4/3 inches, or 39.375, or 39.6 inches.
6. Measuring the speed of light with a system based on imaginary atoms, lice and barley corns
Would it be possible to measure the speed of light with a unit based on a system that relies on imaginary atoms, lice, their eggs, and barley corns? Well not only would it be possible, but it seems that this was actually done, historically.
There is convincing evidence that it was known in ancient India that light traveled, and there is even evidence that the speed it traveled at was known. Researcher Dr. M. R. Goyal has analysed the units of measure used in ancient India in order to understand a mention in the Rig-Veda of how light travels. The commentary is this: “Salutations to Thee (the Sun) who approacheth (at a speed of) 2202 yojanas in a nimishardha.” (commentary on Rig-Veda, Mandal 1, Sukta 50, Mantra 4, Sayanacharya (14th Century AD)).
It is clear that sun rays are being alluded to, and it cannot be the sun that is travelling towards us, as the earth remains at a more or less constant distance from the sun itself. A yojana is exactly 9 miles, or 190 080 barley corns. A Nimishardha is a unit of time. Dr. Goyal writes:
Clearly it is the Speed of light (or sun-rays) that is mentioned in the shloka. This shloka is attributed[16, pp. 67] to the son of Kanva Maharshi (5th millenium B.C.). To put it in mathematical terms, as stated by the above shloka, the speed of light would be: Speed Of Light = 2202 Yojanas/Nimishardha. As we have calculated earlier, a Yojana = 14.484096 Km. For the value of Nimishardha, we refer to Vishnu Purana (Book 1, Chapter 3, shloka 8,9), wherein it is stated that: 15 Nimishas = 1 Kashtha 30 Kashtas = 1 Kala 30 Kalas = 1 Muhurta 30 Muhurtas = 1 day & night (अहोरात्रम्) Thus, one day & night = 405,000 Nimishas = 810,000 Nimishardhas. (Literal meaning of Nimishardha being half a Nimisha). In Surya Sidhanta (Chapter 1, Shloka 12), it is stated that 60 Nadis constitute one Stellar Day and Night (नाक्षत्रम् अहोरात्रम्). It is also well known that a Muhurta = 2 Nadis. It is clear from this that in Astronomical calculations, the stellar day was taken as the unit of time. A stellar day is the time taken by the Earth to complete a rotation around its axis (with respect to stellar constellations). A stellar day is equal to 23 hours, 56 minutes and 4.1 seconds, or equivalently 86,164.1 sec, as the stellar constellation make 366.25 relative revolutions in a year of 365.25 days. So, Nimishardha = 86164.1/810000 sec = 0.1063754sec The speed of light as given in the Vedic Literature therefore comes out to be: 2202 * 14.484096 / 0.106375 km/sec = 2.998 × 105km/sec which is precisely equal to the speed of light as per the latest modern measurements." (9)
A Yojana is equal to 570 240 inches, which is also 43 200 Saxon / Sumerian feet of 13.2 inches, or 14 400 Saxon wands of 39.6 inches. The speed of light is therefore close to 20 698.044117 yojanas per second. Interestingly, however, the Rig Veda doesn't give the speed in relation to seconds, or some simple division of the day, but in terms of Nimishardhas. According to Dr. Goyal, 1 Nimishardha is 86164.1 / 810000 sec = 0.1063754 seconds. One sidereal day and night are equivalent to 810,000 Nimishardhas.
The Rig-Veda clearly demonstrates that it was known that light traveled, and with a proper analysis of the units of measure mentioned, it is clear that a very good approximation of the speed of light was arrived at. The ancient system that gave rise to this expression for the speed of light should not be taken too lightly. With this in mind, we might give proper attention to other claims or suggestions that perhaps a reference to the speed of light might be made in other legacies from the ancient world. We might also reconsider the need to base the most important unit of the system in use worldwide now, the metre, on the speed of light, which it itself also measures. As Dr Goyal notes:
The Speed of Light as given in the Vedic literature, is precisely equal to the Speed of Light as per modern measurements, which shows that during the period 5000 B.C. to 3000 B.C., Science and Technology was very much advanced, which was somehow lost during the medieval ages.(9)
7. The metre and the day-inch
The metre may be defined today in a less than perfect way, involving speed. It is curious therefore to see that historically the metre seems to have been linked with measures of time, in particular concerning the moon. One of the key old measures it is linked with is the inch.
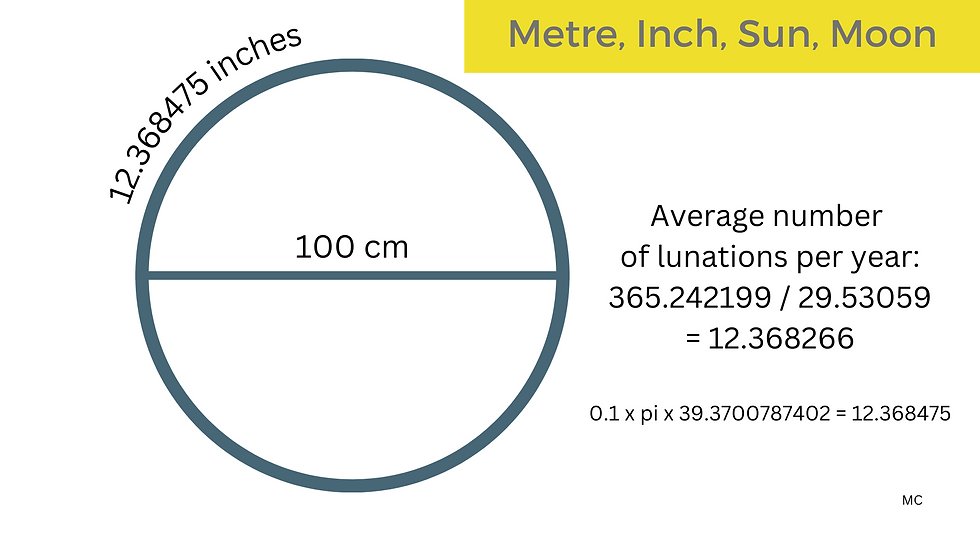
It's possible to think of the metre in relation to "day-inches" in several ways. For example it is linked to the inch via the circle. A circle with a diameter of 1000 x √ / 44 = 39.36479 inches, which is close to the 39.3700787402 inches which define the metre today, gives a circumference of 6 cubits of 20.611356 inches, or 10 units of 12.366814 inches. There are on average 12.368266 lunations in a solar year. If the circle has a diameter of exactly 100 cm, the circumference will be 12.368475 inches, which is closer still tot he average number of lunations.

With 29.53125 for a lunation in days, and 99 / 70 for √2, a circle with a diameter of 12 x 29.53125 inches can be linked to a square with sides of 20 cm. This can also be linked to a Royal Egyptian cubit. A circle with a diameter of 29.53059 x 1 000 inches will have a circumference of 254.0042898 x 365.242199 inches. This is another link between the sun and the moon provided by the inch and the metre, courtesy of the circle.

Also, a circle with a diameter of 35.436708 metres, a reference to the number of days on average in a lunar year, will have a circumference of 365.24836 feet, as discovered by Jim Wakefield.

While the metre can be linked to measures of time, in an ancient context, it seems far-fetched to define it in terms of a speed. Compared to the ancient system, the modern system, at the heart of which is the metre, seems a little unsophisticated, a little simplistic. The fact that the system relies on a a unit that is basically defined by itself doesn't do much to promote it.
8. Conclusion
When the metre was invented, or re-discovered, whatever the case may be, in the 18th century, it became a central part of the revolutionary project. Unfortunately, the main unit of the day in Paris had the word "king" in it, the pied de roi, or king's foot, so there was good reason to find a replacement, in the minds of those who wanted radical change. They could have just changed the name of the pied de roi, which after all had been around for many centuries. Just a few months after the king had been guillotined, a meeting was held to get rid of his foot too, even though the two men in charge of measuring France, Delambre and Méchain, had not finished their task. Before they had returned, the metre had already been instated, albeit a provisional metre.
A revolution is an interesting word to chose for the process of change that took hold of France in the 18th century, as it implies a turn of the wheel. Not only does this suggest the geometry of the circle, but also a connection to the past, and a sense of history in which things can be repeated. There was a keen interest in Ancient Egypt and pyramids in the run up to and in the aftermath of the French Revolution. (14)
The speed of light is a problematic standard for a linear measure. Furthermore, it may not be constant, as pointed out by Norman Wildberger (18) and by Rupert Sheldrake (19) among others.. The metre can historically be linked to both time and space, but whether it should be linked to both at the same time is open to question. If the definition of the metre somewhat undermines it today, it can be partly salvaged by its very long history, in the knowledge, that, way back, it was part of a system which was complex, sophisticated, precise, and which depended on spatial concepts to define spacial units of measure.
Perhaps the use of the speed of light as a standard by which to measure things by is purely symbolic. Light symbolises nature as much as science. The metre and the imperial measures seem to have historical connections to the sun and the moon. The inch, foot and yard don't fare much better than the metre, as they are also dependent on this circularity. Whatever the merit of defining a unit of length in terms of a speed, which makes the standard depend on itself, the image of a light beam drawing out a metre is poetic at least, and sort of futuristic, in an old-fashioned way.
Thanks to Jim Alison for his comments on the barley corn, and the way it is measured.
This post was inspired by Norman Wildberger's video on the speed of light. https://www.youtube.com/watch?v=KiH39mCqnoQ
Notes
Trasareṇu (त्रसरेणु). is also an ancient measure of weight of metals. "jālāntarāgate bhānau yat sūkṣmaṃ dṛśyate rajaḥ / prathamaṃ tat pramāṇānāṃ trasareṇuṃ pracakṣate. //" (Śloka 132, Chapter 8, Manusmṛti). Eight trasareṇus make one Īru. Three īrus make one Maṇkaṭuku. Three maṇkaṭukus make one Veṇkaṭuku. Six veṇkaṭukus make one Madhyastrīyava. Three madhyastrīyavas make one kunnikkuru. Five kunnikkurus make one Māṣa. Sixteen māṣas make one Suvarṇa. This is the weight of two and a half varāhas. Four suvarṇas make one Pala. Ten palas make one Dharaṇa. https://www.wisdomlib.org/definition/trasare%E1%B9%87u#purana The Laws of Manu (c500BC) state: “I will explain, leaving nothing out, the names of (the weights of) copper, silver, and Gold that people normally use to do business on earth.The tiny speck of dust that is seen when the sun shines through a lattice window is said to be the first measurement and is called a ‘quivering atom’. Eight quivering atoms are considered equal to one louse egg, then of these ( louse eggs), three equal one black mustard seed, and three of these ( black mustard seeds) equal one white mustard seed. Six white mustard seeds equal one medium sized barleycorn, and three barleycorns make one berry; five berries make a bean, sixteen beans a gold piece. Four gold pieces equal a straw, ten straws make a support; two berries (of silver), weighed together, equal one silver smallbean. Sixteen of these smallbeans make a silver support or old coin; but, a penny of copper weighing as much as a scratch, is known as a scratch-penny. Ten supports (of silver) should be known as a silver hundred-weight; and four gold pieces are one gold ornament.”
A Likṣā (लिक्षा) is also a measurement of weight, according to the Rasa-darpaṇa (Sanskrit work on rasaśāstra, or Medical Alchemy). Six truṭis constitute one likṣā, and six likṣās constiture one yūkā. https://www.wisdomlib.org/definition/liksha#hinduism
8 Aṅgulas = Prādeśa (?); 21 Aṅgulas = Ratni; 24 Aṅgulas = Hasta; 2000 Dhanus = Gavyūti; | 12 Aṅgulas = Vitasti; 2 Ratnis or 42 Aṅgulas = Kiṣku; 4 hastas = Dhanus; 8000 Dhanus = Yojana. |
4. From the Brahmanda Purana https://www.wisdomlib.org/hinduism/book/the-brahmanda-
5. http://www.individual.utoronto.ca/kalendis/hebrew/chelek.htm Hebrew Calendar Studies:Why Divide Hours into 1080 Parts?
6. Flinders Petrie, M.W. 1911, "Weights and Measures", Encyclopædia Britannica 1911 Encyclopædia Britannica/Weights and Measures - Wikisource, the free online library
7. Ibid
9. Goyal, M.R., “Units of Length Measurement and the Speed of Light in Ancient India”, 18 April 2020, https://www.indica.today/long-reads/length-measurement-and-speed-of-light-in-ancient-india/
10. Heath, Richard, 2012, The Meaning of Le Menec: A Study of the Moon using the Circumpolar Stars https://www.academia.edu/5384849/The_Meaning_of_Le_Menec_A_Study_of_the_Moon_using_the_Circumpolar_Stars
11. See Sir William Matthew Flinders Petrie, 1883, The Pyramids and Temples of Gizeh, https://www.ronaldbirdsall.com/gizeh/
13. See Thom, Alexander, Megalithic Sites in Britain https://archive.org/details/megalithicsitesi0000thom/page/42/mode/2up?view=theater
14. See Howard Crowhurst https://www.youtube.com/shorts/vftB5HX_5JM
16. If the barley corn length is 0.33 modern inches, 8 barley corns, measured by length, will 2.64 inches. The Brahmanda Purana says that 8 grains of barley make an angula. But if the angula is identical to the Sumerian shusi, and 0.66 inches / 1.6764 cm, then 2.64 inches, or even 2.6666 inches, is clearly much too long for the width of a finger. About right for the length... But this doesn't fit with the other values.
According to https://www.indica.today/long-reads/length-measurement-and-speed-of-light-in-ancient-india/]Dr Goya[/url]l "‘Angula’ is considered to be the (average) width of a human finger, because ‘angula’ literally means finger", adding "On analysis of all seven ancient scales found so far – six in India and one in Sumeria (dated 2175 BC) – it is found that all the seven ancient scales are based on the precise value of ‘angula’ as 1.6764 cm. It is further found that the standard street widths of the ancient towns, and also the standardised size of bricks are also based on this unit of ‘angula’, i.e. 1.6764 cm."
If the angula is 0.66 inches, this corresponds to the width of a finger, and so the 8 barley corns must be measured differently. The width of a barley grain is between 0.08 and 0.12 inches, so it is possible to measure the width of 8 barley corns and obtain a length of 0.66 inches.
A louse is supposed to measure between 2 and 3 mm, which is between 0.07874 and 0.11811 inches. If 8 lice make a barley corn and 8 barley corns make an angula, as the Brahmanda Purana suggests, based on the 8 lice alone, not the grain, the resulting finger would be around 5 to 7.56 inches, which is much too long for a width, and even too long for a length of a finger. I measured my fingers and my index isn't even 3 inches long. In any case the angula is supposed to measure the width of a finger not the length. So it must be that the louse in question is a mini louse, of 0.66 / 64 = 0.0103125 inches. Or so I thought till I checked the width of a louse. Width of a louse: approximately 0.04 to 0.12 inches. So I think the lice were lined up side by side and measured by width.
The diameter of a human hair varies but it would be somewhere between 1/1500 and 1/140 inches. Working our way up from this, so multiplying it by 8 four times to get to the angula, is not very helpful, as the hair diameter varies too much, and could produce an angula anywhere between 2.73 and 29.26 inches.
It must be that the angula mentioned in the Brahmanda Purana is measured against the width not the length of the 8 barley grains, and the 8 lice are also measured by width.
If a yojana is indeed 14.484096 km / 9 miles as Dr Goyal suggests, then there is still a problem with the list of units in the Brahmanda Purana, which states that a yojana is 8000 Dhanus and a Dhanus is 96 angulas, which would make the angula 0.7425 inches. So 0.66 inches multiplied by 108 and 8000 (108 instead of 96) do make a yojana which fits with Dr Goyal's estimate. If, however, we start with an angula of 0.66 inches, then multiply it by 96 and 8000, as per the instructions in the Brahmanda Purana, we get 8, not 9 miles.
17. See Jim Alison "Earth Measures", Babylonian and Roman Lengths, Volumes and Weights, https://home.hiwaay.net/~jalison/blu5.PDF




Comments