61. Moon as the Measure of all Things
- M Campbell
- Jun 4, 2023
- 29 min read
Updated: Jan 27, 2024

Protagoras said "Man is the measure of all things". Perhaps he meant that everyone has to perceive, and experience, and figure things out for themselves. Perhaps he meant to remind us that there is no such thing as objective truth. Or perhaps he meant that measuring systems depend on the proportions of the human body. Or maybe he meant that people shape the world we live in, not fate, or gods. When we do try and give shape and order to the world, do we base it simply on abstractions, like number? Or have we historically based it on the very things that govern us, that are way beyond our control, such as the sun and the moon, and perhaps also fate and gods too?
It seems paradoxical that we might design systems of measure and prediction in which to anchor our experiences of the world on the sun and the moon. These two heavenly bodies may be cyclical in their activities, making them suitable for marking the passage of time, but they are also the very things that we have no power over as humans, and on which we depend so much. We experience them through climate and the seasons, light and darkness, the pull of the seas, and even our states of mind. The sun gives us the day and the year as units of time, and the moon gives us the month. As components of the calendar, these periods of time are essential building blocks. Many ancient calendars are lunar, or else combine the patterns of the sun and the moon (lunisolar), and so the moon seems to have consistently had a prominent place in marking the passage of time. Is the moon the measure of all things?
The Latin word for measurement is mensura, and the verb to measure is metiri . Our word for measure is also linked to other languages, such as the Sanskrit mati, the Old Persian ma- , and the Greek metron, also meaning measure. The word "moon" is not only linked to the word "measure", but is also similar across many different linguistic traditions. In Middle English, it's mone, in Old English mona, in Old Saxon and Old High German it's mano, in Old Frisian mona, in Old Norse mani, in Danish maane, in Dutch maan, in German Mond, in Sanskrit masah, in Persian mah, in Greek mene "moon,". The Latin word mensis , meaning"month" also derives from this tradition. And many other languages have similar words for moon and month, such as Lithuanian, with mėnesis "moon, month" Old Irish mi, Welsh mis, Breton miz , all meaning month. The common sound to most of these words is "me", or "ma", or "mo", an etymological root which seems to mean "to measure".
The moon is also connected to intelligence and knowledge in several ancient religions. For example, Manasvini, a Hindu goddess associated with virtue, intelligence is also said to be the mohter of the moon. She is linked by name and with her lunar associations to the Roman goddess Minerva, the wisest of all gods and goddesses. Minerva's name is derived from an older goddess called Meneswā or Menes-wo, meaning “she who remembers,” “she who knows,” or “she who measures.”
The moon's motion is an ancient widespread measure of time. It's no surprise that the moon's cycles are linked to our calendar months, which have been extended slightly so as to fit within a solar year, but it seems the moon has also been understood as a source of knowledge and even intelligence. Is it possible that the moon may once have provided the foundation for a system of knowledge and measure, designed not just for calendars, but for all things? One way to find out is to look at ancient sites and see if numbers associated with the moon's cycles occur there regularly enough to be able to make a connection between the moon and the measure of both time and space.
Lunar numbers
So what numbers qualify as lunar? The number of days in an average lunar month, 29.53059, is an obvious contender, followed by the number of days in a draconic month, 27.2122, and in a draconic or eclipse year, 346.620075883. The moon's sidereal rotation period is 27.321661 days. There's also the number of lunar months in a Metonic cycle of 19 years, 235, as well as the number of lunar months in an 8 year Venusian cycle, 99. Other cycles include a 223 lunar month period, which Halley named Saros. There's also 12 and 13, the number of possible months in a solar year, and the average number per year is 365.242199 / 29.53059 = 12.3682662. The number of days difference between the solar and lunar years is 10.875119. 309 lunations are 25 years of 365 days, a cycle the Egyptians were fond of. As Guyla Priskin has observed, 309 + 309 = 618, that's a good approximation of 1000 phi. Then there's 7 and 4, the number of days in a week, and weeks in a month. And there are other less obvious numbers, like 47. Numbers that already depend on a system of measure, such as the dimensions of the moon, are also important. 1080 is an example, with the equatorial radius being 1,738.1 km, which is 1080.005 miles. Perhaps it is too wild to imagine that the mile was designed so as to be able to express the moon's radius with a number that was already central to a sophisticated way of computing the moon's motion. Why is 1080 lunar?
The Hebrew Calendar

The number 1080 is also central to the Hebrew calendar. In his extensive research on the Hebrew calendar, Irv Bromberg explains that the number 1080 was used to divide time, in particular, the hour, in order to allow the the duration of a lunation to be expressed without use of a fraction. It's quite complicated, but I have quoted Irv Bromberg's explanation to illustrate the sophistication of his ancient calendar and explain some of the numbers involved.
The duration of a part or chelek equals the earlier Babylonian barleycorn (pronounced she), the smallest Babylonian time unit, which was 1/72 of a time degree. The time degree was the principal Babylonian unit of time, corresponding to the time required for one degree of motion of Sun across the meridian = 1/360 of a solar day = 1440 minutes per day/360 time degrees per day = 4 minutes per time degree. Thus 4 minutes divided by 72 = 1/18 of a minute = 1 chelek. The time degree also very nearly equals the difference in duration between the solar day and sidereal day, which in the present era amounts to about 3 minutes and 55.9 seconds. The Babylonian finger was 6 barleycorns = 1/12 of a time degree = 1/3 of a minute = 20 seconds of time. The cubit was 180 barleycorns = 5/2 time degrees = 10 minutes of time. The hour itself, corresponding to 15 time degrees, was a Seleucid time unit that was probably obtained from Egypt. The Babylonian beru or double hour, corresponded to 30 time degrees. The mean synodic month in Babylonian time units was 29 days, 6 double hours, 11 time degrees, and 1 barleycorn.
If we divide the numerator 13753 by 1080 to separate the number of hours from the remaining parts we obtain:
The remaining 793 parts is the same as 2/3 hour + 73 parts = 44 minutes + 1 part = 44 + 1/18 minutes. It is exactly one part greater than 2/3 + 1/15 = 11/15 of an hour.
In one half of the complete molad cycle there are 25920/2 = 12960 months, so each complete cycle contains an excess of 13753 – 12960 = 793 full months, which corresponds exactly to the remaining 793 parts in excess of 29 + 1/2 days. Likewise there is a deficiency of 12960 – 12167 = 793 deficient months, since that number of otherwise deficient months are made full in each complete cycle.
Therefore the traditional molad interval is 29 days, 12 hours, 793 parts. The duration of the molad interval is critical to the traditional Hebrew calendar arithmetic, and must be expressed using only whole numbers and proper fractions so that any date can be calculated exactly and unambiguously. (Long after posting this web page, I learned that the Vilna Gaon similarly explained that only the division of hours into 1080 parts allows the duration of the lunation to be expressed without use of a fraction, see the Kol Eliyahu commentary on Talmud Bavli tractate Rosh HaShanah page 25a.) Exact calculation of a molad moment isn't as complicated as it might seem, because, as pointed out by Rambam (chapter 6) the 29 days comprise 4 weeks plus 1 day remainder, therefore if one already knows the molad moment for a given month then the molad moment of the next month will be 4 weeks, 1 day, 12 hours, and 793 parts later. Similarly, if one knows the molad moment for Tishrei of a given year (used in determining the date of Rosh HaShanah), then the next molad of Tishrei after a non-leap year will be 12 × (4 weeks, 1 day, 12 hours, and 793 parts) = 354 days and 9516 parts = 50 weeks, 4 days, 8 hours, and 876 parts (73/90 of an hour = 48+2/3 minutes = 48 minutes and 40 seconds) later, and after a leap year will be 13 × (4 weeks, 1 day, 12 hours, and 793 parts) = 383 days and 23269 parts = 54 weeks, 5 days, 21 hours and 589 parts (32 minutes and 13 parts) later. (3)
The number 54 is half of 108, and these numbers are both also associated with the precessional cycle, and with Indian astronomy. So it's interesting to see a period of 54 weeks here.
Giza

A good place to start looking for lunar numbers in buildings of the ancient world is Giza, because it has been precisely measured, and is obviously the work of highly skilled and sophisticated architects and builders. If the three largest pyramids at Giza are considered within a rectangle defined by their relative positions, the dimensions of this rectangle reveal lunar numbers. This rectangle is positioned in line with the north-south axis. Flinders Petrie gives 29,227.19982" (W-E) and 35,713.2” (N-S) as its dimensions. This could be interpreted as follows: the west-east width being 29.53059 x12 x 4 x 20.619 = 29226.8”, encoding 4 lunar years in cubits, or as 365.242199 x 80 = 29219.38, encoding 80 solar years in inches. There is a slight discrepency, as it actually works out as 80.02027 years.
This discrepency is interesting. 80 multiplied by 365.242199 is 29 219.37592, and this, divided by the number of days in 4 lunar years, 1 417.46832, gives 20.613777. As a measure in inches, this is a little shorter than the 20.619" we find by dividing the width by 4 lunar years: 20.61929666 inches. The ratio between these two cubit lengths would be 1.00026777. As a measure in inches, this last figure would give a precise match between 80 solar years and 4 lunar years. The width, as per Flinders Petrie, is 29 227.1998, divided by 80 gives 365.3399975, but if we divide this by the ratio between the two cubit lengths, we get a much more precise correspondance between the length in inches and the length of a solar year: 365.242197 inches.
If the same linear measure can produce 80 solar years in inches or 4 lunar years in cubits, what can this tell us about the relationship between inches and cubits more generally? The ratio between 80 solar years and 4 lunar years is 20.613777. While a little on the small side, this could serve as a measure or an Egyptian cubit in inches. Divided by pi and 10 this gives us 0.656157, which could be a measure or the shusi in inches, which then multiplied by 10 / 9 gives 0.7290362, which could be a measure of the Egyptian digit in inches. The ratio of 80 solar years and 4 lunar years is in fact perfectly representative of the ratio between 20 solar years and a lunar year: 365.242199 x 20 / (12 x 29.53059) = 20.613777.
The north-south length of the rectangle could perhaps be understood as 346.620076 x 5 x 20.6065 = 35,713.13”, encoding five draconic years in cubits, but a much shorter cubit. Another option could be to see this as 1000 x √3 cubits of 20.61929666, found in the width. 1 draconic year is 346.620076 days, and so 5 draconic years are 1733.10038, which is very close to √3x1000, and 4 lunar years in days are reasonably close to √2x1000 days. The √2:√3 theory put forward by John Legon, defining the rectangle as √2:√3, is very intriguing.The length, which John Legon associates with √3, suggests that this irrational number might once have been connected to a period of five draconic years. Another interpretation of the dimensions of this rectangle has been put forward by Jim Alison, Manu Seyfzadeh as 9 x 11 (personal communication). It's a better fit. One possible interpretation of this ratio is that it produces a grid of 99 squares, 99 being the number of lunar months in eight solar years, which also corresponds with a Venus cycle. This theory was also put forward by Don Barone.


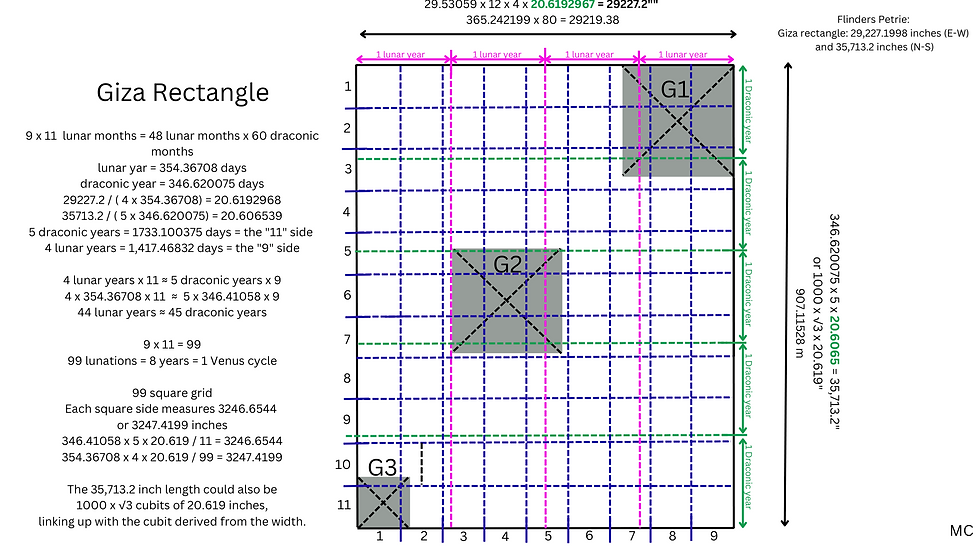
The 99 lunar month cycle which is 8 solar years long defines the Giza rectangle best. The rectangle width can be interpreted both as 80 solar years in inches, and 4 lunar years in cubits of 20.61901 inches. The ratio of 80 solar years and 4 lunar years represents the ratio between 20 solar years and a single lunar year: 365.242199 x 20 / (12 x 29.53059) = 20.613777. Perhaps the solar / lunar year ratio has simply been applied to the 8 year cycle, and then multiplied by 10, as 80 solar years are 10 Venus cycles, 990 lunations, to a very close approximation.
Further lunar numbers can be found within the overall layout of the pyramids at Giza. For example, a mean side of the Great pyramid is given by Flinders Petrie as 9068.8 inches, and a mean side of the third pyramid, the smallest, is given by him as 4153.6 inches. Multiply these together and you get 3,000 x 29.53059 square cubits, each measuring 20.62". Or as Dennis Payne has suggested this can be further refined to 201.434 x 439.806 / 29.5306 = 3000.003. These are simple linear measures, close to the measures provided by Flinders Petrie. What might the significance of 3,000 lunations be?
The traditional figure given to the length of a precessional cycle in years is 25,920. The relation between the solar and lunar years can be expressed as pi multiplied by the length of a precessional cycle divided by 30,000. So, with 22/7 for pi, and the number 10.875119 as the difference in days between twelve lunar months and a solar year, 3,000 x 10 x 29.5306 / 10.875119 x 7/22 = 25,919.992. The length of a lunation has gradually decreased over the last few millennia, and the length of a solar years has increased slightly, so this 10.87512 number may not correspond exactly to the number used long ago by astronomers, but must be close. Perhaps this equation was known long ago.
The width of the Giza rectangle can be thought of in terms of lunar month, because 295.3059 x 11 x 9 inches gives a figure quite close to the width of the Giza rectangle. And 295.3059 x 11 x 11 matches the length of the Giza rectangle reasonably well, with the first number being about 8 inches longer than Flinders Petrie's measurements, and the second about 18.8 inches longer.
Dennis Payne has also found that if we take the measure between the third pyramid and the Great pyramid, centre to centre, we obtain a measure of 36 864 inches. Using a measure of 9071.8 inches for the side of the Great Pyramid, we can divide this by 36 864, and then multiply by 120, which gives 29.5306.

Another example of a potential lunar code is in inches, in the dimensions of the Great Pyramid itself. The height is given as 5776" by Flinders Petrie, and this could be interpreted as √(365.242199 / 354.36708 x 20,000,000 x Phi) = 5775.267, involving the solar and lunar years in days and the golden ratio. The slope of the pyramid then becomes √(365.242199 / 354.36708 x 20,000,000 x Phi²) = 7,346.26086 inches, and the base becomes √(365.242199 / 354.36708 x 10,000,000 x Phi x π). This means that the main dimensions of the pyramid's exterior could be understood as expressions of the ratio between the solar and lunar year, in inches. The ratio between a solar year and lunar year that can be found in the 20.613777704 inch cubit returns here, and perhaps refers to the solar and lunar values found in the Giza rectangle itself. (365.242199/(29.53059 x 12) x 20 = 20.61377705)
A surprising lunar correlation appears when a 52 week calendar, which belongs as much to the cycles of the Maya and Aztecs as to the modern calendar, is applied to the Great Pyramid base. If we take the 9068.8 inch per mean side of the Great Pyramid, as per Flinders Petrie, and mulitply it by 4 to get a base perimeter of 36 275.2 inches. Converted to metres, this is 921.39008 m. Divided by 52, this is 17.71904. Multiplied by 10, this is the number of days in 6 lunar months. Divided by 6, this figure gives a lunar month of 29.5317333, which is very close to the current estimate of 29.53059. It would be a perfect match if we reduced slightly the figure for the mean base side to 9068.448888 inches, which is well within Flinders Petrie's parameters. If the perimeter of the Great Pyramid corresponds to 6/10 lunar months, in modern metres, then one side can be thought of as 29.53059 days, a lunation, multiplied by the number of 7 day weeks in a calendar year, 52, multiplied by 3/20, equals 230.338602 metres, or 9068.448888 inches. Coincidentally, 230.34 is the number of inches in the height of the King's chamber, as pointed out by Dennis Payne, and so the same lunar associations can be made there, but in inches instead of metres. Stephen Dail has observed that Petrie noted the use of a cubit of 20.607 inches in the height of the King's Chamber, and that this cubit corresponds to the cube root of 8750, which, multiplied by √125, gives 230.39326 inches.
Yet another example is in the relative layout of the two largest pyramids. The N-S distance between their south faces is 346.32 metres, and the N-S distance between their centres is 353.68 metres.
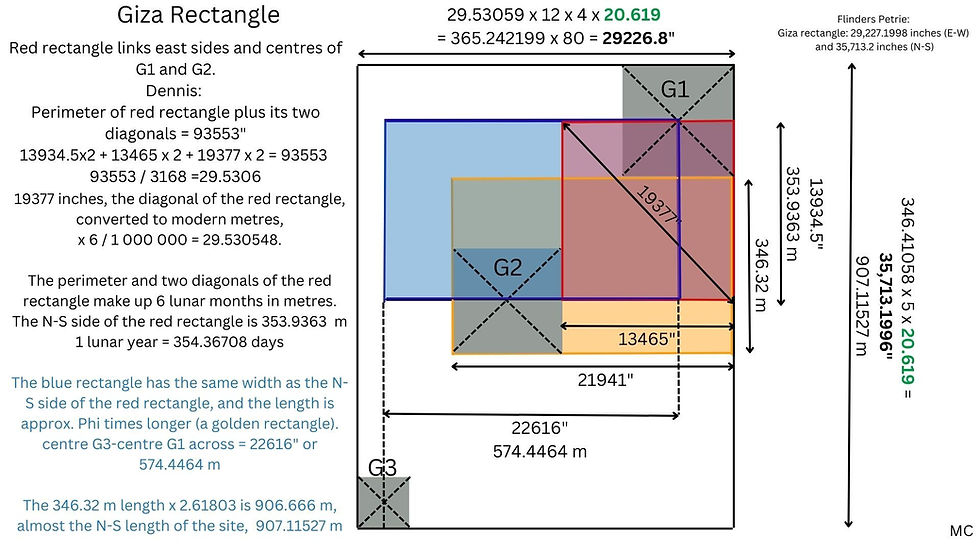

The image below shows possible lunar interpretations of the dimensions of the rectangle formed by the positioning of the three pyramids. One side of the Great Pyramid multiplied by one side of the Menkaure pyramid, in cubits, produces 3000 lunar months. (439.821 x 201.442) = 29.53059 x 3000.225. One side of the second pyramid can be interpreted as 411 cubits, 411 being a lunar cycle in days.

The diagram below shows a possible understanding of the dimensions of the great pyramid in terms of the ratio between the solar and lunar years, combined with the golden number, Phi.


The diagram below is an illustration of a discovery made by Jim Wakefield while looking at the dimensions of another pyramid, the glass pyramid in the Louvre.

This diagram is an illustration of the connection of the metre and the inch in terms of the sun and the moon. What if the metre were understood as an expression of the lunar month in inches, multiplied by some factor? Perhaps the metre could be thought of as 29.53059 x 4 / 3 inches, or 47 x 4 / 15 x π inches? This would produce a value for the metre of 39.374628 inches, which is not far from 39.375". The metre, as expressed in inches, is close to the ratio between the solar year and the lunar month, x 10/π. Jim Wakefield has found that 9 metres x 39.375 is 354.375.

This diagram is about how the number of days in a lunar month can be squared, and multiplied by pi and 25, to produce the number of days in a Metonic period of 19 years. The number of months in a Metonic period can be arrived at by multiplying the number of days in a lunar month by 25 and dividing by pi. As a result you could express pi itself in lunar terms, for example 29.53059 x 25 / 235, or 29.53059 x 29.53059 x 25 / (365.242199 x 19). You could interpret the length of one side of the Great Pyramid of Giza, given by Flinders Petrie as 9068.8 inches, as 29.53059 x 29.53059 x 4/3 x 78/10 = 9069.38 inches, which is just over an inch over 29.53059 x 78/10 metres (of the modern kind). In fact, 29.53059 x 4/3 = 39.3812 inches, which is just over a modern metre in inches, of 39.3700787402 inches.

Brilliant examples of the moon at Giza and other ancient sites have been found by researchers such as Jim Wakefield and Dennis Payne, as below. Dennis Payne has found some amazing lunar connections, many of them at Giza. Below are three images created by Dennis, published on Academia, in which he explains the connections he has found, at Giza. the diagrams speak for themselves. This work is absolutely precise, and suggests a total re-think of how we understand this ancient site.
The first diagram presents the number 47. This number is explored further below.
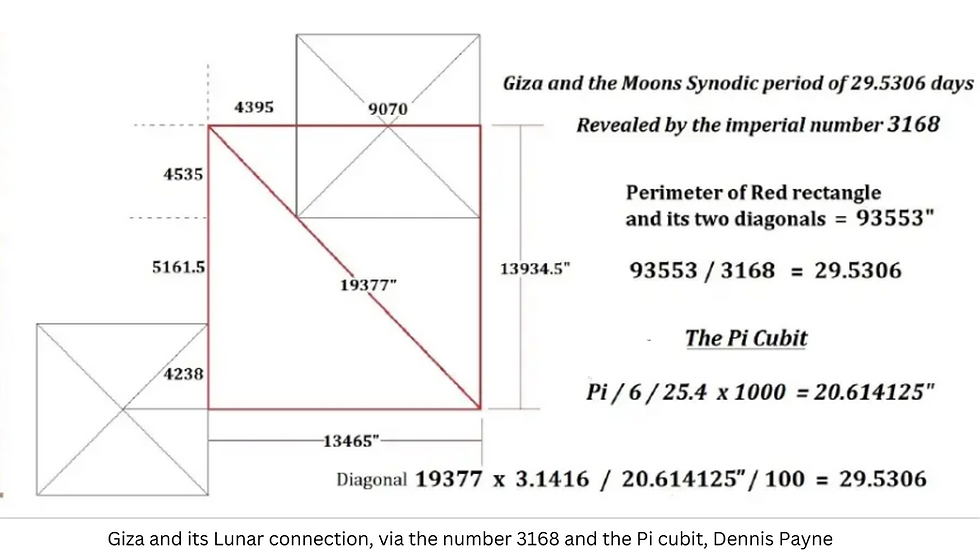
In the diagram below, Dennis shows a geometric Lunar correlation in the distance between the two larger pyramids at Giza. The diagonal of the red rectangle is 19377 inches. Converted to modern metres, multiplied by 6 and divided by 100, this is 29.530548 m. So the perimetre and two diagonals of the red rectangle make up 6 lunar months in metres. The number 254 links the metre to the inch. However, the diagonal can also be interpreted as solar, in that it is 365.242199 x 1000/6π = 19,376.7 inches.
The triangle below is particularly impressive, and brings up the 29.53059² number that was seen in the Metonic period, above.

As Dennis has pointed out, "if we accept the premise of turning constant numbers into linear units of measure, then one has to accept they did have a unit equivalent in length to our modern inch."
John Michell, in his book Ancient Metrology, uses a ratio to convert the common cubit of Samos to the Egyptian Royal Cubit which may be lunar, and this ratio is used also by Jim Alison and Stephen Dail. The Earth/Moon distance varies between 407,000 km (apogee), and 357,000 km (perigee). The ratio between the perigee and apogee figures can be simplified slightly to 22/25. A 12 inch foot x 126/125 = 12.096, and this is the Samos foot. This Samos foot multiplied by 1.5 to make the cubit, and then by this 25/22 ratio gives 20.61818 inches, which is one possible value for the Egyptian Royal cubit. with this particular cubit, the Great Pyramid's base side, taken as 9072 inches, measures exactly 440 cubits.
John Michell also came up with this well known equation: the canonical earth radius, 3960 miles, plus the canonical moon radius, 1080 miles, are 5040 miles, 5040 being an important number for Plato, in the Republic, and equal to 7! (which is 1 x 2 x 3 x 4 x 5 x 6 x 7). Taken as a radius, this 5040 multiplied by 2 and 22/7 for pi, produces a circumference of 31680 miles, and this is yet again another important number, and equal to 4 earth diameters of 7920.
A close examination of the dimensions of the pyramids and their relative position reveals a simple progression from 10 000 inches can explain many of the dimensions, as illustrated by this diagram. In particular the number of days in a lunation, 29.53059, is a factor that links various sections of the Giza plan, together with pi and the square root of three. In fact, the number of lunations in a year, on average, can be approximated by this fraction: π x √3 x 100 / 44 = 12.36681. So that even pi and the square root of three can be considered lunar. The 440 cubits present in the mean base side of the Great Pyramid can also be related to this equation. And the mean base side of the Great Pyramid can be understood as π x √3 x 10 000 / 6 = 9068.9968 inches.
By multiplying the number of days in a Sothic year by the number of days in a Draconic year, and 3√3, then dividing by 20π and the number of days in a lunation, 29.53059, we can obtain approximately the number of days in a lunar year. Perhaps this why we find the square root of 3 and pi in various proportions at Giza.
In the image below, the number 10 001.4822 has been plugged into an algorithm, which seeks to approximate the dimensions of key parts of the Giza plateau through simple numerical connections. 10 000 would work also as a starting point, but 10 001.4822 works better, suggesting that if the inch was used at Giza it may have been very slightly different to our modern inch.

Stonehenge
Here is a diagram of one of Jim Wakefield's findings at Stonehenge:

Jim shows that the Sarsen circle's dimensions can be connected to the lunar cycle.
The diameter at Stonehenge is close to the circumference at Stanton Drew, where a similar comparison with the moon can therefore be made.
Teotihuacan
Dennis Payne has found intriguing lunar connections at Teotihuacan too. The circuit around the Citadels platform is 4677.646 ft, which, divided by 1584, gives 29.53059. In inches, this becomes 316.8 x 6 x 29.53059, or 1900.8 x 29.53059. 19008 and 3168 are important numbers at Stonehenge, with 19008 being 11 x 12³. This perimeter could also be interpreted, as Dennis does, as 504 metres of 39.375", with 504 being 12 x 6 x 7.

In the image below, Dennis presents more measurements from Teotihuacan. Of interest here is that the total number of feet he arrives it could be interpreted as close to 1900.8 x 29.53059 / 12 = 4 677.64, though it is just over a foot longer than 4 676.53.

In the following image, Dennis shows that the number 47 and the Moons period of 29.531 days, encoding Pi, can be seen in the dimensions of the platforms of the Citadel. Dennis writes:
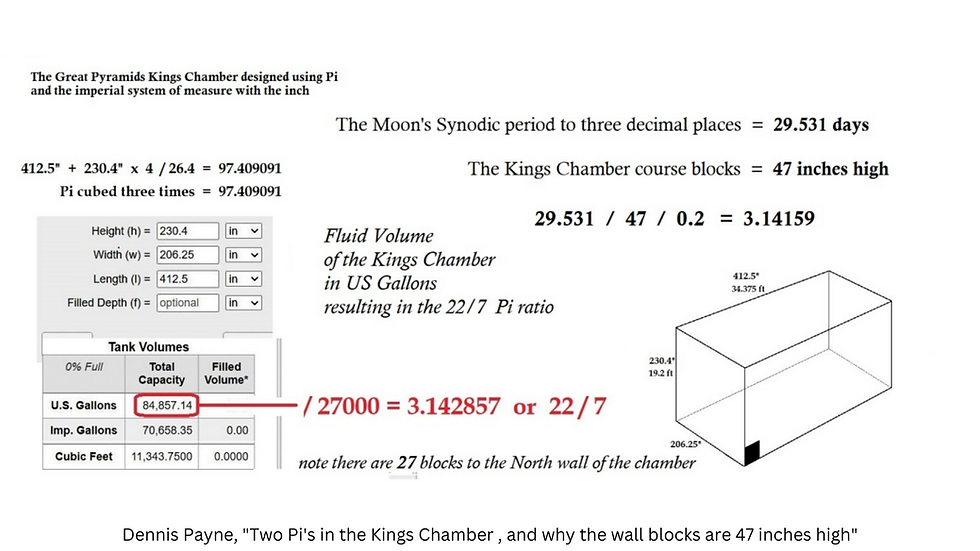
If we accept the idea that these platforms have been set out in such a way as to encode certain sets of information, then it would be important to get an accurate determination of how they relate to each other via their measured distances , in this case I am referring to the distance from each of the lower four platforms to the central platform of the Plaza, and again I am using the imperial system of measure to uncover what may be hidden. As you can see from the screenshot , I have measured the four distances from the lower platforms to that central one. The four combined = 1566.67 ft and x 3 = 4700 ft. Then the Moons cycle of 29.531 / 4700 / 2 = 3.141596. The number 47 has also been found in the Great Pyramids Kings Chamber, in that each course is 47 inches high.

The number 47
Dennis Payne discovered a lunar connection to the number 47, as shown in the first diagram of his posted here, and Jim Wakefield has also found this number in connection to an ancient site and the moon.
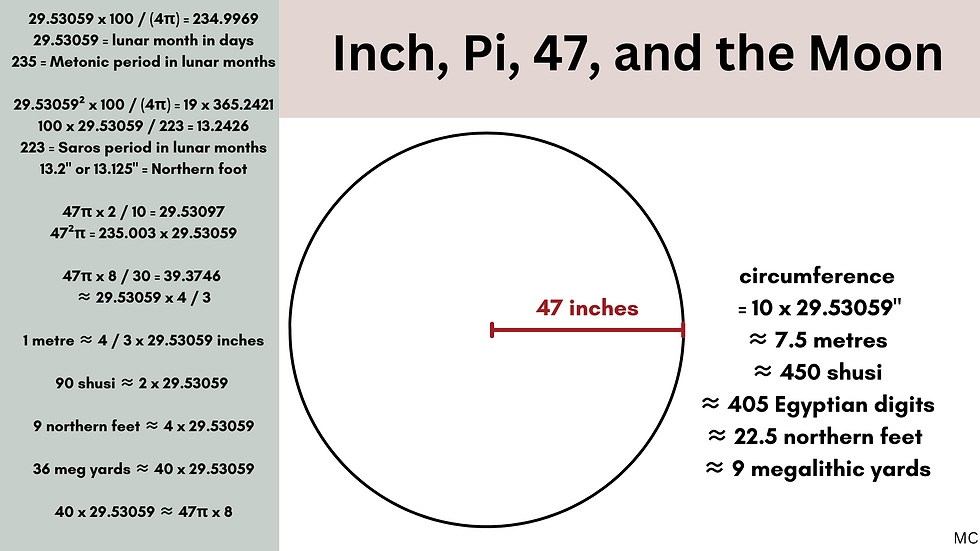
Jim Wakefield discovered a curious metrological fact at the Rollrights stone circle: that the number 47 is a lunar number. As we've seen, there are 29.53059 days in an average lunar month, and 12 to 13 lunar months per year, and there are cycles of lunar months which coincide with cycles of solar years, such as the Metonic cycle of 19 years, or 235 lunar months. The number 47 is simply 235 x 2 / 10. What Jim found was that a circle with a radius of 47 units will have a circumference of almost exactly 10 lunar months expressed in the same unit. That is, 47 x 2 x pi = 29.53097 x 10.
Tthe other lunar aspect of a circle with a radius of 47 units, which Jim found: that the area of such a circle will have almost exactly the same number of units as there are days in a Metonic cycle.
Metonic cycle: 29.53059 x 235 = 6939.68865
area of circle with radius of 47 units: 47² x pi = 6939.77817 units squared
"Metonic" pi could be defined as 365.242199 x 19 / 47²
The unit that this was expressed in at the Rollrights was the Northern or Saxon foot of 13.2 inches. Jim writes about:
the coincidence of the Rollright circle having a diameter of 94 Saxon feet and therefore a circumference of '295.309 Saxon feet equaling the number of days in a synodic month 29.53059 days and then the area of the circle having 6939.778 square Saxon feet equaling the number of days in a metonic cycle 6939.75 days (1)

The Saxon foot is an ancient unit, usually thought of as 13.2 inches, with direct links to India, as 13.2 inches are 200 Angulas of 0.066 inches. In 1930-31, at Mohenjo-daro, Ernest Mackay discovered a broken piece of shell bearing 8 divisions of 6.7056mm each, which is equivalent to 0.264 inches. A Saxon foot is 50 x 0.264 inches. 96 Angulas make an ordinary Dhanusha, and this is exactly a thousandth part of an English mile. The links between the English and Indian measuring systems go way back in time.
It is all the more curious that the number 47 works so well within a circle to describe ten months and a Metonic cycle through the circumference and area, when the number of months in 2 Metonic cycles is 470. Without invoking any circles, the number 47 connects the solar and lunar cycles: 38 years of 365.242199 days are almost exactly equal to 470 lunations of 29.53059 days. Furthermore, 38 x 2 / 47 provides a good approximation of the almost Phi relationship between the sun and moon cycles, as 76 / 47 = 1.61702127 and 29.53059 x 20 / 365.242199 = 1.6170415. The average number of lunations per solar year can be thought of as 47 x 20 / 76. A year in days is very close to 29.53059 x 20 x 47 / 76. The metre as a measure in inches relates to the cycles of the sun and moon, with 39.3700787402 x pi / 10 is equal to the average number of lunations in a year. The metre in inches multiplied by 76 x pi / 200 is very nearly equal to 47.
As illustrated earlier, Dennis Payne has also discovered the connection between the lunar cycles and the number 47 at Giza: inside the King's Chamber, in the Great Pyramid, the height of the course blocks is 47 inches.
Piazzi Smith and Petrie both agree that the course heights of the Kings chamber are 47 inches high, with a tiny + - of 0.040 One must wonder why these blocks were made to that height, if one believes they only used cubits for their measurements then what would the stone mason make of being asked to make these blocks 2.279340446 cubits high, assuming they used that well known cubit of 20.62". (2)
Is the number 47 an important part of historical metrology more generally? Perhaps this link between the circumference and the area of a circle, expressed through pi and the number 47, has a wider application?
Irv Bromberg has another connection between the number 47 and the moon. He writes:
There are 235 months per 19-year cycle of the Hebrew calendar. The divisors of 235 are 1, 5, 47, and 235, whereas 19 is a prime number. The average number of months per traditional Hebrew calendar year = 235/19, so the number of years in the molad moment repeat cycle = 25920 / (235/19) = 2095+31/47 years. Every 18 months the molad moment is exact to the minute with zero parts remaining. Every 1080 months or 87+7/22 years the molad moment is exact to the hour with zero minutes and zero parts remaining, which happened most recently for the traditional molad moment of Cheshvan in year 5765. A full cycle of every possible molad moment landing on every possible weekday takes 7 × 25920 = 181440 months / (235/19) = 689472 / 47 = 14669+31/47 years (this is to be expected because 181440 is the number of parts per week). A full cycle for the molad of Tishrei of the first year of the 19-year cycle to go through every possible molad moment landing on every possible weekday takes 47 times longer, or 689472 years. (3)
Irv Bromberg has remarked:
There are 235 months per 19-year cycle of the Hebrew calendar. The divisors of 235 are 1, 5, 47, and 235.
He explains what the molad interval in the Hebrew calendar is, and finishes by saying:
A full cycle for the molad of Tishrei of the first year of the 19-year cycle to go through every possible molad moment landing on every possible weekday takes 47 times longer, or 689472 years.
For all these reasons, it is clear that 47 is an important lunar number. There is another cycle associated with the number 47 and it is connected to Mars. Mars returns to the same position relative to the earth and sun after 47 years, or 22 x 780 days. This cycle was known in ancient Mesapotamia.
47 is also the atomic number of silver, a metal associated with the moon.
Dennis Payne has found that the number 47 also connects the speed of light with the equatorial radius of the earth. So the number of times light might, if it could, travel through to the centre of the earth from the equator in a second is 47.003139 (using 186 282.39 mps and 3963.1906 miles), and 47 x π x 2/10 - 29.53097, and 47 x 47 x π = 6939.778, number of days in a Metonic cycle, and 47 x 10/2 = 235, the number of lunar months in Metonic cycle, plus there's the connection between 47 and the calculation of the molad.

You could think of the speed of light as:
29.53125 x 400,000,000 x 1224/1225 inches per second, using 29.53125 as the length in days of a lunation. Even with the correct lunation mean, 29.53059, you still get 299,785,871.3022 metres per second, still close to the official 299,792,458 mps.
Curiously, the Moon is gradually moving away from the earth, into a higher orbit, and researchers have calculated that in about 50 billion years, the earth and the moon will be tidally locked, and the moon's orbit will have changed from 27 to 47 days. Also, the angle of the precession of the equinoxes cycle measured from the centre of the earth has been valued at 47 degrees.
Stephen Dail has used 47 to express the lunar diameter values. 47 is 22 + 25, and these two numbers are numerators of two Pi approximations, 22/7 and 25/8, involved in so many of his calculations, as well as his figure for the ApoTerrian of 25 units, and Periterrian of 22 units of the Moon's eccentric orbital cycle. The value 47 / 2 = 23.5 becomes the semi major axis of the Moon's mean distance from the Earth in it's monthly orbital cycle, also being the canonical distance mentioned by John Michell.
A year can be defined with the help of the number 47, as 153 / (47 x 28) x 1000 x pi = 365.245954. The 153 is found in the biblical story of the fish in the net, and 28 is lunar in that it is 4 weeks of 7 days. So a circle witha diameter of 153 x 1000 /(47 x 28) would have a circumference of 365.245954. If we replace the number 1000 in this sum with the number of days in a lunation, this gives us approximately the difference in days between a solar and lunar year: 153 / (47 x 28) x 29.53059 = 10.785929 and 365.242199 - 354.36708 = 10.875119. Also of interest is 153 x 10 000 / (28 x 47) inches are 29.530395 metres. We might even think of the metre as 153 x 10 000 / (28 x 47 x 29.53059) = 39.3698189 inches. We might even think of the Metonic cycle as 153 x 100 000 / 56 inches converted to metres: 6939.64286 days.
There is also an interesting connection to the megalithic yard, as part of a vesica piscis, as below.

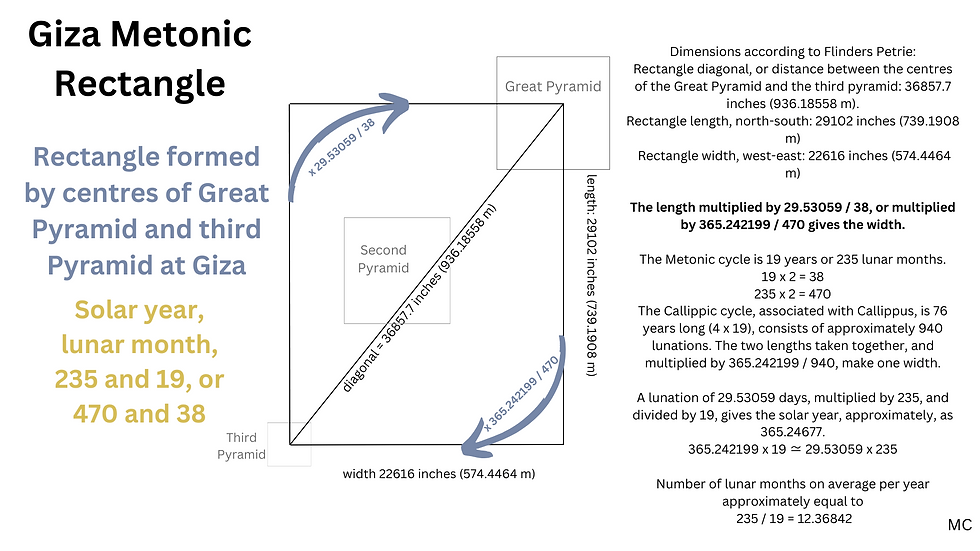
At Giza, Flinders Petrie gave the length of the distance between the centres of the Great Pyramid and the third pyramid as 36857.7 inches, which is 936.18558 m. If this line is the diagonal of a rectangle, the length, north-south, is 29102 inches, or 739.1908 m, and the width, west-east, is 22616 inches, or 574.4464 m. If we take the width and multiply it by 29.53059, the number of days in a lunation, and 38, we get the length. And conversely, if we multiply the length by 365.242199, the number of days in a year, and divide by 470, we get the width again. It's a Metonic rectangle.
22616 x 38 / 29.53059 = 29102
29102 x 365.242 / 470 = 22616
The number 47, combined with the number 38, is present in this Giza rectangle, which stretches out between the centres of the Great and third pyramid at Giza. A lunation of 29.53059 days, multiplied by 47 x 10, and divided by 19 and 2, gives the solar year, approximately, as 365.24677. This means the number of lunar months on average per year can be simplified as 470/38, which is 12.36842 and 365.242199 / 29.53059 = 12.368266. This solar and lunar ratio is slightly different to the one found at the Rollrights by Jim Wakefield, yet it also uses the number 47. At the Rollrights, the radius of a circle was 47 Saxon feet, and the circumference was approximately 10 lunar months in days expressed as Saxon feet. At Giza, we have a rectangle, and the ratio between the width and length is 38 / 29.53059, to within about half an inch: which is roughly equal to 365.242199 / 470. The width of 22616 x 38 / 29.53059 = 29 102.29697. The length as given by Flinders Petrie is 29102. This 29102 multiplied by by 365.242199 / 470 = 22 615.47379, which is 0.5262 inches less than Flinders Petrie's figure.

Here we can see that two lunar rectangles can be seen to define the space at Giza, and they are lunar not by virtue of any particular unit of measure, but in their proportions.


A system of measure based on the moon?
We can write a lunation in days as approximately 47/5 x π, or 94π/10, and so intricately associated with the geometry of the circle. What if the metre were understood as an expression of the lunar month in inches, multiplied by some factor. Perhaps we could think of the metre as 29.53059 x 4 / 3 inches, or simply 47 x 4 / 15 x π inches? This would produce a value for the metre of 39.374628 inches, which is not far from 39.375 inches, a historical value, or from the current value of 39.3700787402 inches. And if we can interpret the height of the Great Pyramid as 10 000 / √3 inches, we can also say it is 37 500 / (47 x π x √3) metres.
Perhaps we can also think of the shusi, the megalithic yard, and the northern or Saxon foot, which Jim Wakefield found at the Rollrights, as expressing lunar numbers in inches, multiplied by a simple factor.
The metre, as expressed in inches, is close to the ratio between the solar year and the lunar month, x 10/π. The connection between the metre and the inch could perhaps also be understood in terms of the sun and moon. 1000 x pi lunations are very nearly 254 years, and 1 metre is 1/254 inches.
The role of astronomy in the history of measure is contested. There isn't much in literature to attest to astronomy being used a s a foundation for systems of measure, which is why the findings at Giza and at megalithic circles are so intriguing. It seems that when you look to the stones directly, and their measurements, there may well be signs that the cycle of the moon, and its relationship to the sun are in fact central to ancient metrological systems.
This connection may well also be found in modern day systems of measure, though whether it is there by design or by coincidence is an open question. For example, there is a link between the mile and the cycles of the sun and the moon.
Below are images to illustrate these connections.






If, at Giza, the same linear measure can produce 80 solar years in inches or 4 lunar years in cubits (the west-east rectangle side), what does this tell us about the relationship between inches and cubits? The ratio between 80 solar years and 12 lunar years is 20.613777. Divided by pi and 10 this gives us 0.656157, which could be a measure or the shusi in inches, which then multiplied by 10 / 9 gives 0.7290362, which could be a measure of the Egyptian digit in inches. A cubit of 20.61377 inches is also very close to pi/6 metres. You would obtain a metre of 39.36941 inches 20.613777 x 6/pi = 39.3694141
864
864 is also exactly one thirtieth part of the traditional figure given to the length of a precessional cycle in years, 25,920. The solar and lunar years, and the wobble of the earth’s spin on its own axis, are all connected to the number 864. The relation between the solar and lunar years can be expressed as pi multiplied by the length of a precessional cycle or Great Year (traditional value) divided by 30,000. If one thousand lunar months of 29.53059 days and the number of days difference between the solar and lunar years, 10.875119, then divided by pi as 22/7, gives 864, then 3,000 lunar months give, in the same way, the precessional cycle. 30,000 x 29.53059/10.875119 x (7/22) = 25,919.983, call it 25,920. Michael S. Schneider points out that 86,400,000 is a very nice number. He asks:
How many milliseconds are in a day?
Obviously, 1000 milliseconds/second x 60 seconds/minute x 60 minutes/hour x 24 hours/day = 86,400,000
or, more neatly = 5⁵ x 4⁴ x 3³ x 2² x 1¹
The average difference in days between the lunar year and the solar year, 10.87512 days, in terms of pi, or at least, an approximation of pi: one way of expressing the average difference between lunar and solar year is with 19/7, or more precisely, 19.008 / 7, which can also be expressed as 864 x 22 / 7,000, which is close to 864 x Pi. The 22/7 fraction is thought to have been a commonly used approximation of Pi in antiquity, useful for preserving integers in the measures of diameters and circumferences of the same circle, and a sort pragmatic solution to the problem of incommensurability between diameter and circumference that ‘true’ pi presents. It’s use has been deduced from studies of circular layouts in monuments from ancient times. The number of days in a lunar month divided by the difference in days between twelve lunar months and a year is therefore very close to Pi as 22/7 times 0.864. Twelve lunar months of 29.53059 days give a lunar year of 354.36708 days. That’s 10.875119 days fewer than a solar year of 365.242199 days. You could say that pi itself can be approximated as 1 lunation / difference in days between twelve lunar months and a year divided by 0.864.
29.53059 / 10.875119= 2.7154268
864 x 22 / 7,000 = 2.71542857
The number 864 can be associated with lunar and solar years. A circle with a diameter of 864 units would have a circumference of approximately 1,000 lunations / the difference in days between solar and lunar years. By coincidence, the sun has a diameter of 864,000 miles. So you could say the sun has, in miles, a circumference of 1,000,000 lunations / the difference in days between solar and lunar years.
The lunatic fringe
If nothing else, these lunar findings are intriguing. Were the lunar numbers deliberately inscribed into the Great Pyramid and megalithic circles? If so, they demonstrate incredible sophistication and complexity. The motion of the moon is potentially a way to track time but also measure space too. The numbers associated with the moon are not just the ones that deine the sidereal, calendar and draconic months. There are several important cycles involving the moon. Furthermore, lunar numbers may not necessarily be connected to a cycle, but rather to geometry which ties various elements of these cycles together, as with the numbers 47 and 864, and even pi. Conversely, the moon can be used to express these cyclic and geometric patterns.

Notes
1. Wakefield, Jim, "From the Rollrights to Stonehenge"
2. Payne, Dennis, "Two Pi's in the Kings Chamber , and why the wall blocks are 47 inches high"
(10) Two Pi's in the Kings Chamber , and why the wall blocks are 47 inches high | Dennis Payne - Academia.edu
3. Irv Bromberg, Why Divide Hours into 1080 Parts? (utoronto.ca)
Bibliography
Alison, Jim, Proposed Design Specifications for the Great Pyramid and for the Siteplan of the Giza Plateau.
Bromberg, Irv, Why Divide Hours into 1080 Parts? (utoronto.ca)
Michael S Schneider, www.constructingtheuniverse.com
Payne, Dennis, "Two Pi's in the Kings Chamber , and why the wall blocks are 47 inches high"
Papers by Dennis Payne:
(10) Two Pi's in the Kings Chamber , and why the wall blocks are 47 inches high | Dennis Payne - Academia.edu
At the Citadel the number 47 , the Moons Synodic period of 29.531 (rounded) and Pi at 3.141596 | Dennis Payne - Academia.edu
Schneider, Michael S., 1995, A Beginners Guide to Constructing the Universe, Harper Perennial, New York
Wakefield, Jim, "From the Rollrights to Stonehenge"




Hello Melissa Campbell,
I would like to talk with you privately with you about one thing of your blog.
Write me here: guillermioguillermo64@gmail.com
The duration of a part or chelek equals the earlier Babylonian barleycorn (pronounced she), the smallest Babylonian time unit, which was 1/72 of a time degree. The time degree was the principal Babylonian unit of time, corresponding to the time required for one degree of motion of Sun across the meridian = 1/360 of a solar day = 1440 minutes per day/360 time degrees per day = 4 minutes per time degree. Thus 4 minutes divided by 72 = 1/18 of a minute = 1 chelek.
The chelek must be 0.003858025 inches if the above is to hold against the 13.2 inch lunar standard of Lagash
One minute is then 0.069444444 inches
60 minutes one hour 4.166666667 inches
24 hours…
Melissa again you have hit it out of the park with this Blog , and i am honoured to have some of my work included into your blog, many thanks , when is the Book coming out .