
Jim Wakefield has written a fantastic paper on the Rollright Stones, in relation to an analysis of the circle in Saxon feet, an old unit of measurement also known as the Sumerian foot, worth 13.2”. He writes:
A circle has a centre and a circumference, and the distance between is the radius. The radius of the Rollright Stones circle is 47 Saxon feet and that fact, to me, is a coincidence, because it produces these remarkable numbers:
• the circumference of the Rollright Stones circle = 295.309 Saxon feet
• the number of days in a synodic month = 29.5306 days
• incredibly, the area of the Rollright Stones = 6939.778 square Saxon feet
• the number of days in a metonic circle 6939.75 days.(5)
This is an incredible find, as it means that the number 47 multiplied by 2 and pi gives the number of days in 100 lunar months approximately, and 47 squared and multiplied by pi gives the number of days in 235 lunar months, which is a Metonic period, also approximately. So a circle with a radius of 47 units, of whatever kind, is lunar in character.
In the book The Lost Science of Measuring the Earth, co-written with John Michell, Robin Heath writes:
The English Foot is made to represent the difference in time between the lunar and solar year (10.875119 days) (4)
Why are numbers so closely related to the moon's cycles to be found in Saxon feet, defining a megalithic stone circle? Why is a unit of measurement itself seemingly derived from the moon's cycle? What is the moon's role in measuring our world?
Robin Heath and John Michell showed, in The Lost Science of Measuring the Earth, that at some point before 3,000 BCE, the inhabitants of the British Isles knew the dimensions of the Earth, and had accurately surveyed at least parts of Britain and Ireland. They were using units of measurement derived from the cycles of the sun and the moon and the size of the Earth. They also showed other connections between places which imply advanced mathematical and surveying skills, and seem to be compatible with various aspects of the sun and moon. This was what first ignited an interest for me in making connections between certain places, with the lunar and solar cycles. John Michell wrote:
The key is in number. All the ancient units relate to each other, and to the dimensions of the earth, by the same code of number as is found in every other ancient form of art and science.(1)
For me, the magical part of what Robin Heath and John Michell had shown in their analysis of solar and lunar cycles represented in units of length, such as the foot and the Astronomical Megalithic Yard, was not only that solar and lunar cycles were probably used to calibrate units of length, but that important places were built in relation to others using significant numbers of these units to position them. I'd like to explore the idea of the moon having a key role in these numbers, particularly in units of length, and in spatial arrangements of important sites across the surface of the planet.

The Revelatio is a manuscript which describes the 8th century Bishop of Avranches’s experience in planning out the new abbey to be built at the Mont Saint-Michel. It shows how troubled he was about where exactly to position the building and what dimensions it should have. It describes how he had a vision or a dream instructing him. “It was told to the bishop: "Go, and where you will see the sign, lay the foundations."(2) This illustrates an old belief, not just a Christian one by any means, and perhaps not altogether outdated, that sacred buildings should be built according to special designs. This would include the use of special units of measurement, and of special locations, and the placing of the structure according to specific parameters, in order to reflect in every way something of the divine. This must derive from a world view in which the divine is all around, in every thing, and the entire cosmos is present in the smallest part, and is concentrated in certain places and in certain ways. The divine itself may be understood as a creator god, or company of gods, or as the divine within the world itself, as in pantheism, or perhaps Buddhism or Shinto. This view may go back a very long way. One way of truing to understand the people who built the oldest structures on our planet is to study their measurements and geographical location in relation to each other and key landmarks.
The study of ancient metrology, ancient sites and ancient calendars has revealed that long ago measures relied upon fairly sophisticated mathematics, and interpretations of several astronomical cycles. Researchers in ancient metrology have demonstrated the great antiquity of some units common to us today, and their link to the cycles of the sun and moon, and to the size of our planet. (1) This begs the question: how did the people who originally come up with these measures know the size of the planet and exact length of the astronomical cycles they seem to be derived from? Perhaps most intriguing of all is that the system that units of measurement belong to are part of a single worldwide system which has come down through the centuries. John Neal also says:
“It was simply by comparing the foot lengths of the different national systems that a very elegant order was perceived, leading to the conclusion that all of these “national systems” formed a single organisation. It has become customary for us to name a unit from the society in which it is found to have been used, but most often, the bureaucracies have adopted particular units during the historical period. Obviously a universal system had been fragmented into these various cultures.” (3)
Proportion is also important, irrespective of the units used, and can also reveal a connection to the moon. Robin Heath’s lunation triangle is defined by Stonehenge, Lundy Island, and the bluestone site, where some of the stones that make up Stonehenge are thought to have come from, in the Preseli hills, in Wales. It is exactly 2,500 larger than the station stone rectangle at Stonehenge. The proportions of both the Lunation triangle and the station stone rectangle are 5:12:13.
He calls the triangle a geometrical device for predicting the moon’s phases and eclipses, and in that respect, the unit of measurement it’s in is irrelevant, this is purely to do with proportion. Basically you can make a 5:12:13 triangle quite simply with a length of rope, marked at equal intervals to create thirty equal spaces. Then you can peg it down so as to have 5 on one length, 12 on the next, and 13 on the last. That last and longest length is key: pick it up again and bring it down to divide the triangle into two parts. If you position the third, longest length so that it bisects the shortest side at the 2/3 point, this creates a new smaller triangle, with a hypothenuse of 12.369 units. This represents the length of the solar year calibrated in lunar months or lunations. The average number of lunations in a solar year is 12.368267. Of course, it's difficult to see how such a system, if rolled out across hundreds of miles, can be of any practical use.

Robin Heath writes:
If the English Foot is made to represent the difference in time between the lunar and solar year (10.875119 days), then:-
The megalithic yard, based on the astronomy of the lunation cycles of 29.53059 days, becomes 2.71542857 feet in length.
And: foot : megalithic yard = differential : lunation period.(4)
The average difference in days between the lunar year and the solar year being 10.87512 days, Heath comes up with this: one lunation in days divided by these 10.87512 days is 2.715426, which is close to 19/7, and that's 2.7142857, and both 19 and 7 are numbers associated with moon cycles, the week and the Metonic cycle (of 19 years). So one way of expressing the average difference between lunar and solar year is this number, 19/7, or to fit into the system more precisely, 19.008 / 7, which can also be expressed as 864 x 22 / 7,000, which is close to 864 x Pi. (And in fact 22 / 7 was a commonly used approximation of Pi in Antiquity, as has been deduced from studies of circular layouts in monuments from ancient times.)
As the solar and lunar calendars are not exactly aligned, it’s helpful to think in terms of average number of lunations per solar year. So, there are on average 12 lunations plus 7/19 of a lunation in a solar year. Derived in another way again, you could say that the difference between the length of the solar and lunar years is 10.875199 days (365.242199 – 354.367 = 10.875199 ) and this can also be (just about) expressed as 7/19 of a lunation, or 29.53059 x 7/19 = 10.879691, which is very close to 10.875199. Robin Heath points this out: 7/19 = 0.36842 and 19/7 is 2.7142857. Far from being just a logistical headache for calendar designers long ago, it seems that the difference between the solar and lunar calendars could have been understood as a fascinating, perhaps sacred thing, the expression of which can be seen concentrated within units of time, and even in units of space. It is the numerical marriage of the two most important heavenly bodies for our planet, moon and sun being the main sources of light, and apparently equal in size due to a quirk of distance and actual size. This ratio is used as a starting point for carving up space and time in a systematic manner.
This ratio between two different time cycles becomes a a number of inches, to form a spatial unit of measure. I am tempted to suggest a comparison with the idea of light, in particular, the speed at which it travels, linking units of time and space. The speed of light used as a constant converts time units (like seconds) into space units (like metres). Seconds multiplied by meters/second = metres. The sun and the moon contribute directly to the conversion of time units to spatial units, just by emitting (or reflecting, in the moon's case) light.
The numbers associated with the sun and moon cycles, as well as their size, form a system that is at the base of our measurements today. These numbers are not necessarily the exact expressions of a cycle, but sometimes a close approximation, which fits in well with other numbers. For example, 360, 30, 12, and 6.
There are 40 of these units in the shorter side of the 5:12:13 Station Stone rectangle at Stonehenge. It is worth noting that the numbers 864, 22 and 7 are all important numbers in trying to understand the very old systems of marking space and time. The numbers 22 and 7 combined provide an approximation for Pi, as 22/7. Seven is a number that comes up time and time again, 7 colours of the rainbow, 7 notes in a musical scale, the storm ends on the seventh day in flood legends, etc. And 19 is significant because there is another cycle of the moon which lasts almost exactly 19 solar years: the Metonic. This is the shortest amount of time into which you can (pretty much) fit an integer number of lunations into an integer number of solar years. As a matter of fact, the number 19.008 can also be used to define the ratio between lunar and solar cycles: there are an average of 12.368267 lunations per solar year, or 13 lunations minus 0.631733 of a lunation each solar year, or close to 13 lunations minus (12.008/19.008 of a lunation) in a year.
Also, Robin Heath points out that the two principle circles at Stonehenge have diameters that form a ratio of 7/19, or always very close to that (within 0.5%). (2)

You don’t have to accept Robin Heath’s hypothetical AMY unit to accept a link between the mile and the cycles of the sun and the moon. One lunation in days, 29.53059, divided by the average difference in days between the lunar year and the solar year, 10.87512 days, multiplied by 7,000/36, is 5,279.996. There are of course 5,280 feet in a mile, so if you take this to be a value in feet, it’s very close to a mile.
In fact:
5,280 x 10.87512 x 36 / 70,000 = 29.5306
And so one mile, in feet, can be defined as 70,000 lunations / (36 x the difference in days between solar and lunar years).
Consequently, earth’s equatorial circumference in feet
= 70,000 lunations x (√(π3 x 20,000,000) / ( 36 x the difference in days between solar and lunar years)
= √(10,000,000 π) x √2 x π x 5,280
and the equatorial circumference of the earth in miles
= 365.242199 x 1,296 x the difference in days between solar and lunar years / 7 lunations
the meridian circumference of the earth
= 1752 x 1,000,000 lunations / ( 3 x the difference in days between solar and lunar years x 176) inches
This means that the foot and the mile may be derived from the relationship between the solar year and twelve lunar months. (The 10.87512 is the difference in days between the solar and lunar years, and the 29.5306 is the number of days in a lunation, or lunar month. The length of a lunation has gradually decreased over the last few millennia, and the length of a solar years has increased slightly, so this 10.87512 number may not correspond to the number used long ago by astronomers.)
When Robin Heath looked at the distance between Stonehenge and the island of Lundy, he found a distance of 864/7 miles separated them. Lundy - Stonehenge, is simply 864/7 x 29.5306 x 70,000/(10.87512 x 36) feet, or 240,000 x 29.5306 / 10.87512 feet.
Lundy - Stonehenge distance in feet = 240,000 lunations in days / the difference in days between a solar and lunar year. If you apply this logic to the Stonehenge - Mont Saint - Michel distance, which is almost equal to the Stonehenge - Saint Michael's Mount distance, the result is:
Mont Saint-Michel - Stonehenge distance in feet = (2,400,000/7) lunations in days / the difference in days between a solar and lunar year.

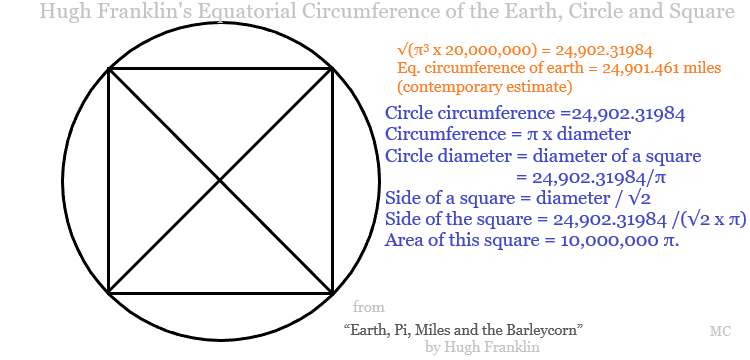
Hugh Franklin's idea of the mile being derived from the equatorial circumference of the earth is very intriguing. It's based on the idea of the diameter (or a part of a diameter) of a circle creating a square or a circle, and a square which has the same area as a circle. In his article “Earth, Pi, Miles and the Barleycorn”, he points out that the number 24,902.31984, which is pretty much the equatorial circumference of the earth, is in fact √(π³ x 20,000,000) (he doesn't quite put it this way in fact, but I think this is easier). So if you have a circle with a circumference of 24,902.31984, and the diameter of this circle is also the diagonal of a square, the square will have side of 24,902.31984 /(√2x π), and the area of this square will be 10,000,000 π. This square would be equal in area to a circle with a diameter of 10,000,000.
This would suggest that the equatorial circumference of our planet, via the geometries of the circle and the square, gave rise to the mile itself, as a unit of measure. The circumference of the earth as a value in miles is equated to a square with an area of 10,000,000 π. The circumference and the area of a circle with a diameter of 1 is π.
So, approximately, one mile = earth’s equatorial circumference /( √(π³ x 20,000,000)
= √(10,000,000 π) x √2 x π /earth’s equatorial circumference
With 1 lunation as 29.53059, and the difference in days between the solar and lunar years as 10.87512 days, you can take one mile, in feet, as approx. 70,000 lunations / (36 x the difference in days between solar and lunar years),
then earth’s equatorial circumference in feet
= 70,000 lunations x √(π³ x 20,000,000) / ( 36 x the difference in days between solar and lunar years)
= √(10,000,000 π) x √2 x π x 5,280
Which is √(10,000,000)×√(2)×π×√(π) miles.
This suggests a diameter of √(20,000,000×π) miles.
And the equatorial circumference of the earth in miles
= 365.242199 x 1,296 x the difference in days between solar and lunar years / 7 lunations
the meridian circumference of the earth
= 175x 175 x 10,000,000 lunations / ( 3 x the difference in days between solar and lunar years x 176) inches
You could even use a pi as 1,000,000 lunations / (difference in days between solar and lunar years x diameter of sun in miles), so 29,53059 x 1,000,000 / (10.87512 x 864,000), which is pretty much 22/7.
Here are some possible lunar connections I have found in relation to Saint Michael's Mount and the Mont Saint-Michel: The number of miles between Saint Michael’s Mount and the Mont Saint-Michel may correspond to the ratio between twelve lunations and a solar year times two hundred. There are 206.14 miles between these two rocks, centre to centre. 365.242199 / 354.367 x 200 = 206.1378. I looked at other moon cycles and wondered how they might fit in. I found that the full moon cycle, the time it takes the sun, as seen from Earth, to complete one revolution with respect to the perigee of the moon’s orbit, is 411.784 430 29 days. Divide this figure by two and you get 205.8922. This figure can be measured in miles between the Mont Saint-Michel and Saint Michael’s Mount.

22/7 is an approximation of pi. The number of days in a lunar month divided by the difference in days between twelve lunar months and a year is therefore very close to Pi as 22/7 times 0.864. Twelve lunar months of 29.53059 days give a lunar year of 354.36708 days. That’s 10.875119 days fewer than a solar year of 365.242199 days. You could say that pi itself can be approximated as 1 lunation / difference in days between twelve lunar months and a year multiplied by 0.864.
29.53059 / 10.875119= 2.7154268
864 x 22 / 7,000 = 2.71542857
The number 864 can be associated with lunar and solar years. A circle with a diameter of 864 units would have a circumference of approximately 1,000 lunations / the difference in days between solar and lunar years. Funnily enough, the sun has a diameter of 864,000 miles. So you could say the sun has, in miles, a circumference of 1,000,000 lunations / the difference in days between solar and lunar years.
Jean-Sylvain Bailly wote that the ancients divided the degree into 24 fingers, or digits, and that they gave 12 of these to the diameter of the sun and of the moon as they appear to us in the sky - and they usually appear to be the same roughly size. He considers the cubit a measure of a degree in the sky, and says that the Chinese only had 10 digits per cubit and also 10 digits per celestial degree, so there must be a connection bewen cubit and degree. 24 digits multiplied by 360 are 8,640, which is a solar number, and associated with the diameter of the sun in miles. So you could consider the number of cubits in the horizon in cubits 4320, half the diameter of the sun in miles and twice the diameter of the moon in miles.
Bailly also notes that Diodorus of Sicily writes about a gigantic measuring instrument, which would have been a huge circle, like an artifical horizon. It was found in the tomb of a king called Osymandyas, in Luxor / Thebes. One cubit corresponds to a day in the year.
Quote Diodorus of Sicily, Book I, 49, 3-50 In a circle about this building are many chambers which contain excellent paintings of all the animals which are held sacred in Egypt. There is an ascent leading through these chambers to the tomb as a whole. At the top of this ascent there is a circular border of gold crowning the monument, three hundred and sixty-five cubits in circumference and one cubit thick; upon this the days of the year are inscribed, one in each cubit of length, and by each day the risings and settings of the stars as nature ordains them and the signs indicating the effects which the Egyptian astrologers hold that they produce. This border, they said, had been plun- dered by Cambyses and the Persians when he conquered Egypt. Such, they say, was the tomb of Osymandyas the king, which is considered far to have excelled all others, not only in the amount of money lavished upon it, but also in the ingenuity shown by the artificers.
On page 179 of his Histoire de l'Astronomie Ancienne, Bailly asks the question: why did the Indians, Chaldeans, Persians, Egyptians all divide things by six?
In all the oldest traditions across the world, the number 60 is associated with counting time, the day might be divided into 60 parts, or there are 60 minutes per hour, 60 seconds per minute.
There's a period of 600 years, or 7,420.9597 lunations, that Cassini found to be very exact, and which the Chaldeans used also. Six hundred is such a lovely round number of years, and this period is very revealing of the importance of the sun and the moon as a team, or duality, that must be reckoned as one. 18 solar years are almost 223 lunar months, 222.62879 to be exact. Three of these cycles, so 669 lunar months, corresponding to 54.090 solar years, or to more exact, 222.62879 x 3 x 29.53059/365.242199 = 54. A cycle of 54 years corresponds to 667.88637, or almost 668 lunations, and this cycle was used to predict eclipses (see Bailly p 140). The number 54 is important in metrology as there are 54 digits in a metre.
However, the the two cycles meet a little more exactly if this period of 222.62879 lunations is multiplied by 100/3, so 7,420.95966667 lunations, almost 7421. This period of 600 years was multiplied by 6 again, for a greater cycle, to make 3600 years. (see Bailly page 110).
It's clear that the numbers 3 and 6 are not just symbolic but practical. They occur often in myths and legends, in relation to time periods. For example the phoenix according to some versions lived for 300 days, and the god Janus is said to have been painted with the number 300 in his right hand and 65 in his left hand according to Macrobius. I think the importance of the numbers 3 and 6 stems from the fact that the cycles they are derived from, to unite sun and moon time periods, is actually very nearly exact, not just an approximation. And it is clear from what is known about very old astronomical conventions that precision was important, and had been acheived. One estimate for the ancient Indian figure for the length of the sidereal solar year is 365.25833333. (see Bailly page 110) In fact, Bailly reports that at his time of writing, the Brahmin tables for predicting eclipses were more accurate than those of the astronomers of his own country.
There are other numbers of course that must derive from the importance of the lunar and solar cycles. For example, seven is perhaps the most important of sacred numbers, and there are seven days in a week, or roughly 7 x 4 days in a lunation. Of course, twelve is very important in imperial measure, and there are between twelve and thirteen lunations in a year. Five is another important number. Very often the year was divided into twelve months of thirty days with a remainder of five days, called epagonomal. The 360 + 5 day year was supposed to be used together with extra days given or taken at set periods, say every 1461 years, to align the calendar with the events of the sky and the seasons. There's also the lunar year 354.36 days, which, like the solar year, could be broken down to a nicer number leaving a remainder of 5, the epagonomal days. Almost everyone in the ancient world seems to have done this, from the Maya and Aztec, to the Egyptians and Babylonians. So 360 + 5, and also 350 + 5(ish) for the lunar year. Interestingly, 360/350 = 72/70.
And yet, it is not necessarily these integers that are the most important in linking units of measure to the cycles of the heavenly bodies, as Plato called them. A stylised lunar month of 29.53125 days converted to chalakim, i.e. the 24 hour period divided into 25,920 parts, in the Hebrew calendar, is 11.666667 x 9 x 9 x 9 x 9 x 10. The Roman foot is 11.666667". The 0.729166667" digit is 29.53125 x 25,920 / (9 x 9 x 9 x 9 x 10 x 16).
This is not a very popular unit with ancient metrologists in th English speaking world (with the exception of Howard Crowhurst, and I think, David Kenworthy and Hugh Franklin), but the modern metre is in fact very interesting in relation to the moon, and the inch. One metre is 1/254 inches, and 254 metres are 10,000 inches. 254 is an important calendar number: there are 29.53059 days in a lunation, but only 27.322 in the tropical month, when the Moon returns to the same spot in the sky (against the backdrop of the same constellation). 254 tropical months are almost equal to 235 synodic months or lunations, the first being 6939.788 days, and the second 6939.691 days. 254 tropical months of 27.322 days. Once again, an important number in aligning the sun and moon cycles, is also important in measure. To keep the moon's cycle as close to the sun's, an extra synodic month and an extra tropical month are added periodically, making a lunar leap year or tropical year. Rather than simply havng 12 synodic months and 13 tropical months in a year, you get instead 13 synodic months and 14 tropical months.
Howard Crowhurst has found that a 3:4:5 triangle can very nicely encompass the numbers 235 and 254: very curiously, if a triangle are these two measures in modern metres for it's longest two sides, the third side will measure 100.003745 x √10 imperial feet. (see Howard Crowhurst's excellent Carnac: The Alignments, Volume 1) This lunar triangle is very intriguing, and I think, suggests the history of the metre deserves to be looked into a little more. Obviously, such a 3:4:5 triangle could also simply be interpreted as 10,000, √85,600,000 and √14,400,000 inches, but the numbers 235 and 254 being such important lunar numers suggest the metre has a role to play.
Also, 254 orbits of the moon are almost exactly 255 lunar nodal months. The Metonic cycle of 19 years is also linked. 254 x 27.322 / 365.242199 = 19.000509. And you can find that 1,000 x 29.53059 x pi / 365.242199 = 254.00407 So 1000 pi lunations are approximately 254 years. I metre in inches is approximately 10 years / pi lunations.

As Robin Heath, Jim Wakefield, and many others have found, it is not just the units themselves of course which may derive from sun and moon cycles, but also the dimensions of sacred places from the ancient world. Remember how the Bishop of Avranches needed some kind of divine guide to help him place and design his new chuch on the Mont Saint-Michel? The Revelatio tells us the the morning dew showed him where to build, but you can be sure he also got more than a little info from his two missionaries he sent to the Monte Gargano site in Italy.
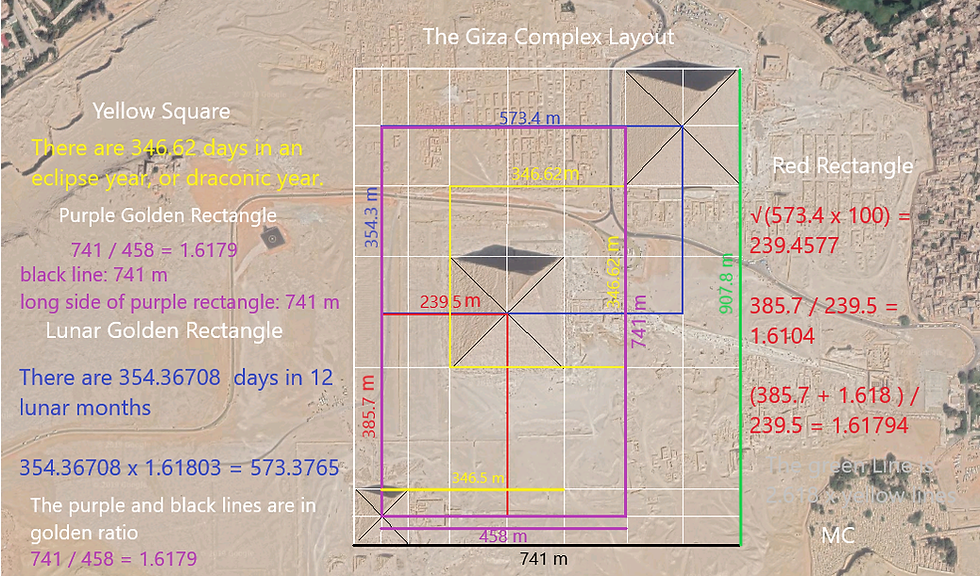
To conclude, here are a couple of examples of possible lunar connections I have found at Giza - though not nearly as many as Dune as found on his threads and here on the GHMB site.

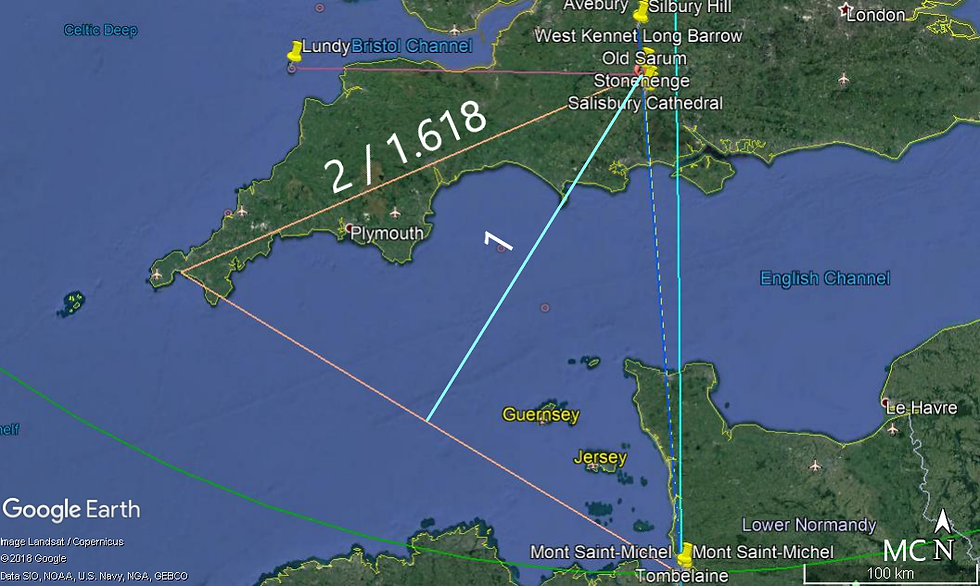
And here, across the sea, between Stonehenge, St Michal's Mount and the Mont Saint-Michel:

Such a triangle is also very nearly a golden triangle.
Notes
Michell, John, 1983, New View Over Atlantis, pp. 125-6
The Revelatio Ecclesiae de Sancti Michaelis and the Mediterranean Origins of Mont St.-Michel, by John Charles Arnold, Department of History, State University of New York-Fredonia, 2007 by John Charles Arnold. All rights reserved. This edition copyright 2007 by The Heroic Age. All rights reserved, in A Journal of Early Medieval Northwestern Europe, Issue 10 (May 2007) | Issue Editors: Celia Chazelle & Deanna Forsman, Saints and Sanctity
" Ancient Measurement Systems: Their fractional integration" by John Neal, 22nd June 2003, July 2003 AOM: - https://grahamhancock.com/ancient-measurement-systems-neal/
4. Heath, Robin, and Michell, John, 2006, The Lost Science of Measuring the Earth, Adventures Unlimited, p. 32
5. “From the Rollrights to Stonehenge—a measure”, Jim Wakefield, Microsoft Word - MEAS-07-Figures_19Sep08_-larger font.doc (dozenalsociety.org.uk)

Comments